| Citation: |
Lin Chen, Jianhua Zhao, Dieter Weiss, Christian H. Back, Fumihiro Matsukura, Hideo Ohno. Magnetization dynamics and related phenomena in semiconductors with ferromagnetism[J]. Journal of Semiconductors, 2019, 40(8): 081502. doi: 10.1088/1674-4926/40/8/081502
****
L Chen, J H Zhao, D Weiss, C H Back, F Matsukura, H Ohno, Magnetization dynamics and related phenomena in semiconductors with ferromagnetism[J]. J. Semicond., 2019, 40(8): 081502. doi: 10.1088/1674-4926/40/8/081502.
|
Magnetization dynamics and related phenomena in semiconductors with ferromagnetism
DOI: 10.1088/1674-4926/40/8/081502
More Information
-
Abstract
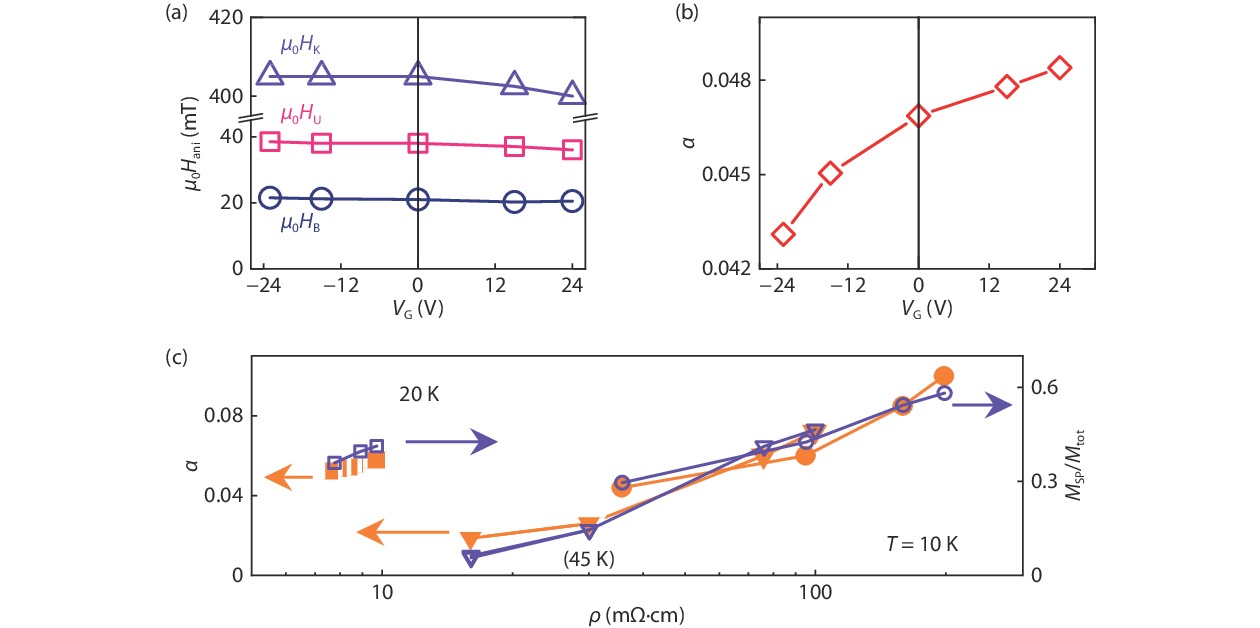
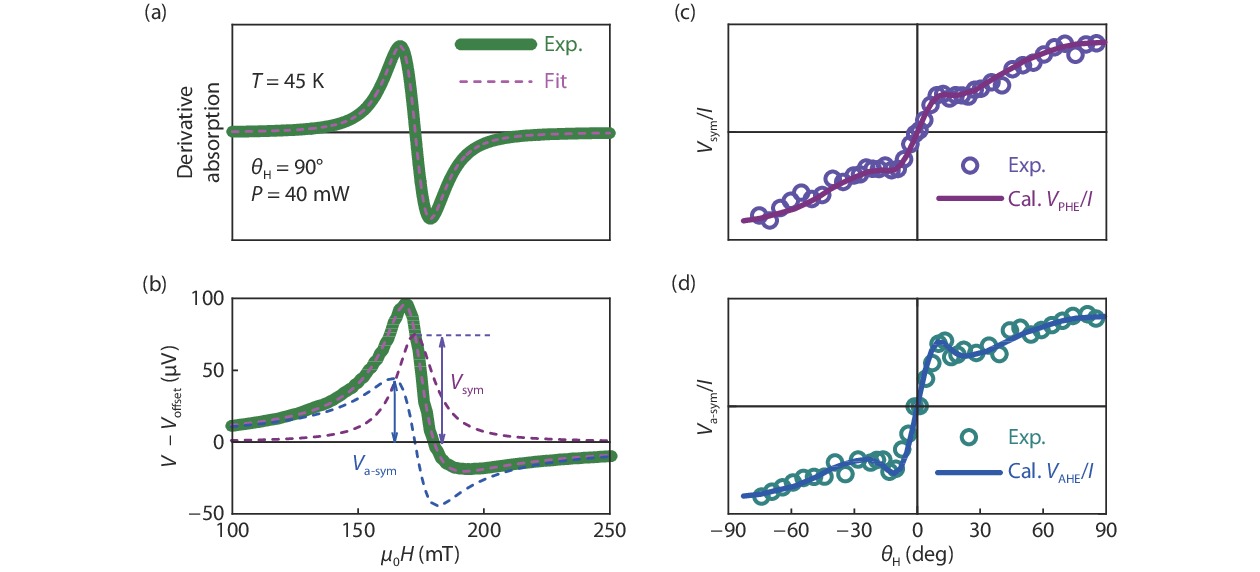
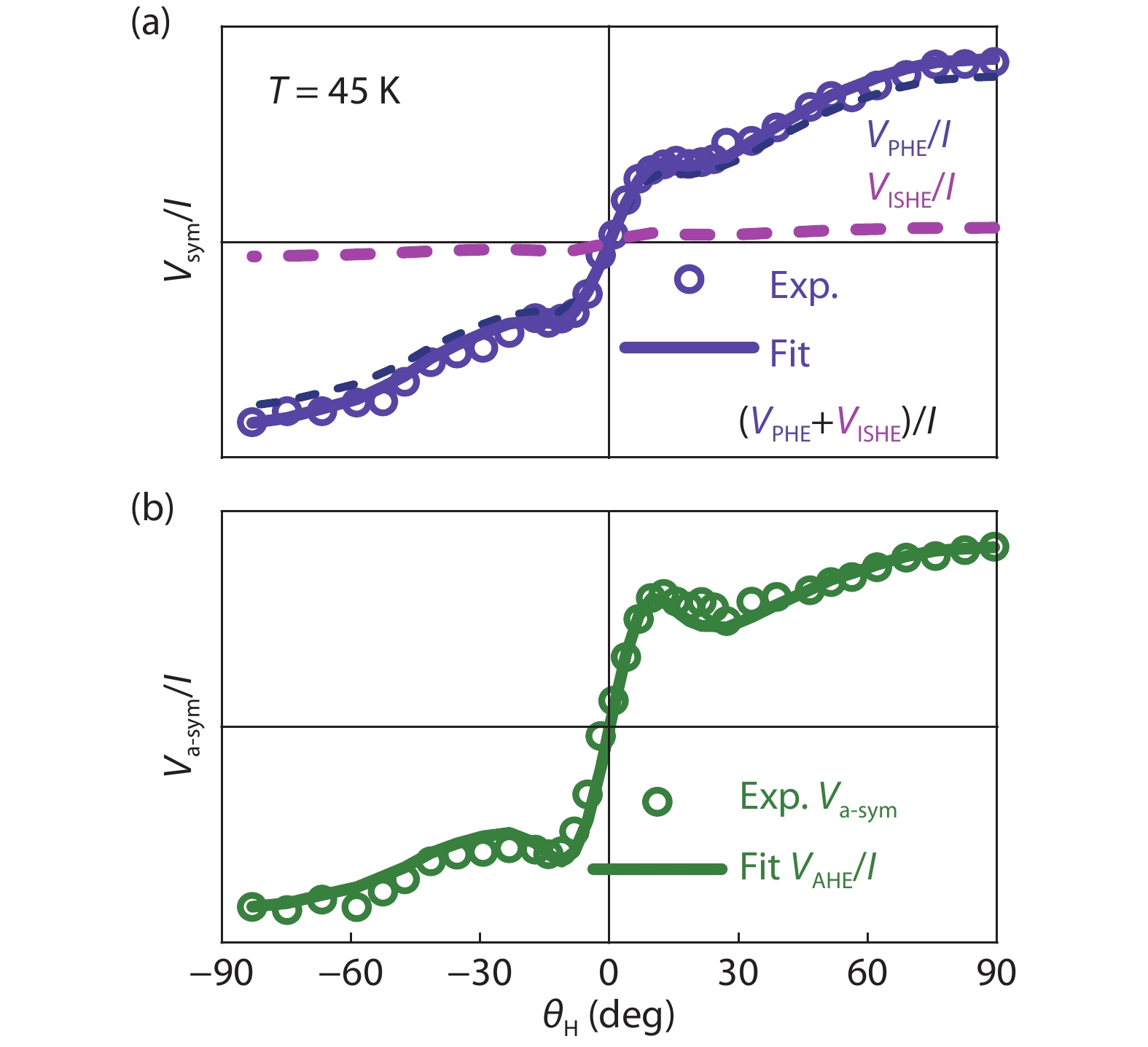
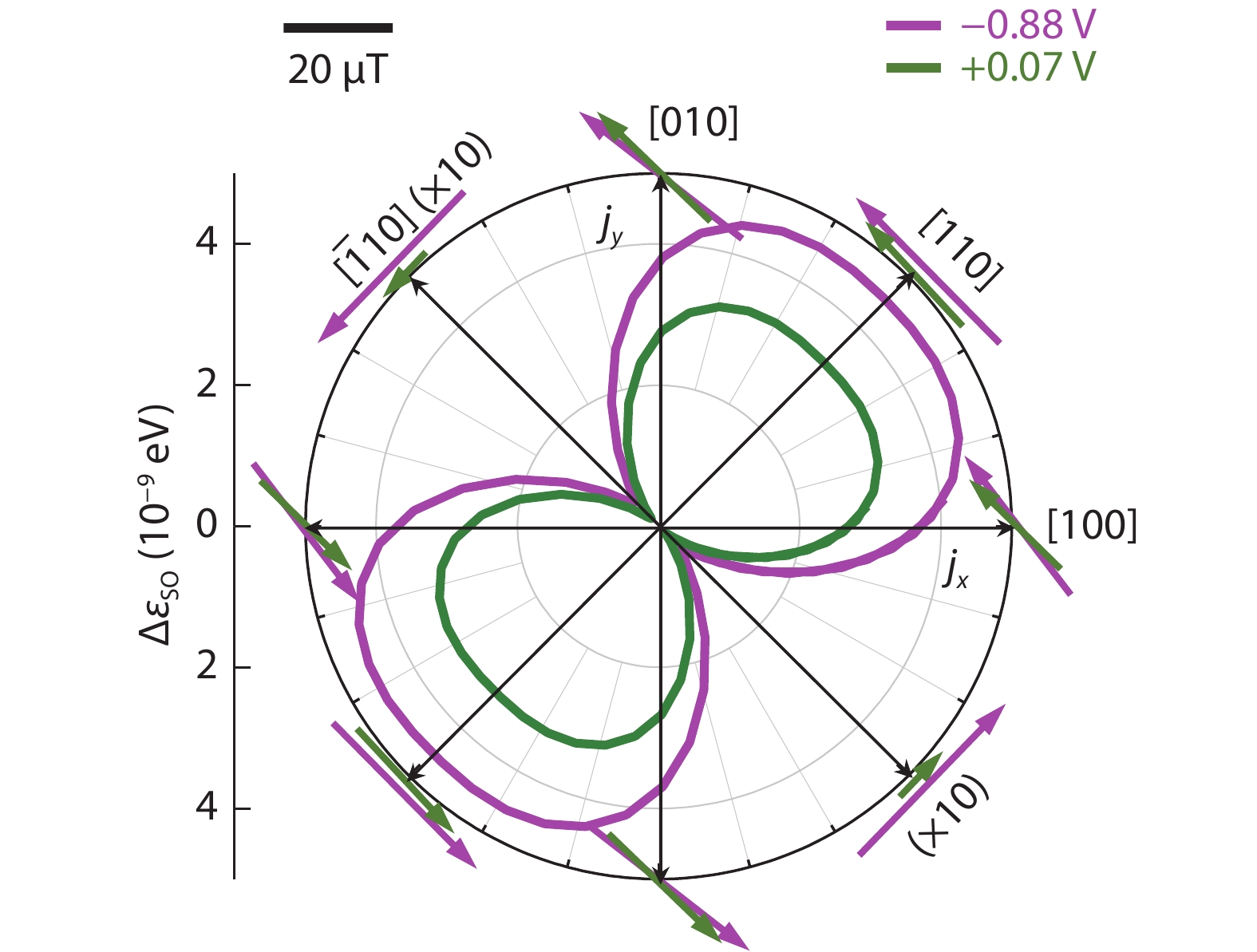
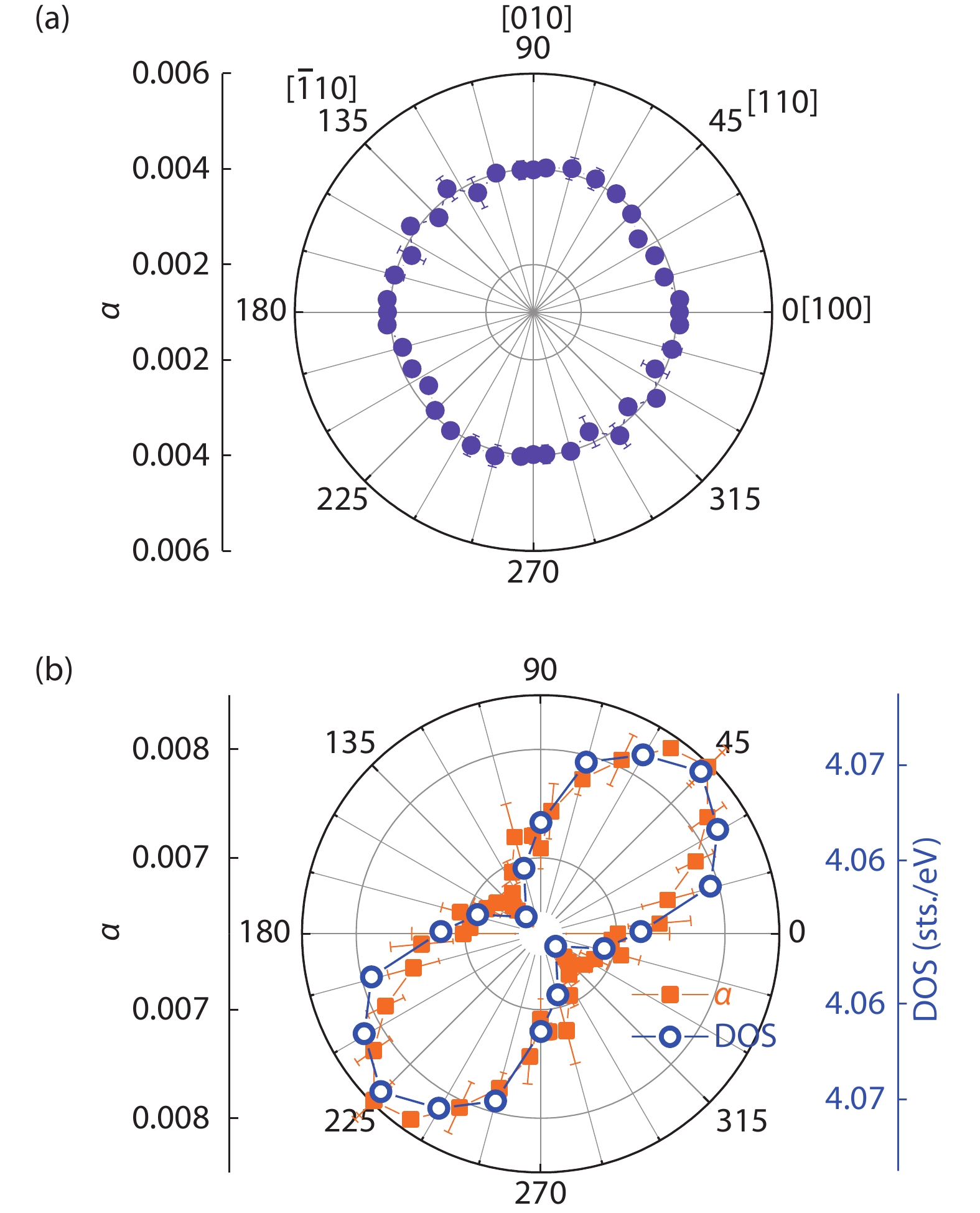
We review ferromagnetic resonance (FMR) and related phenomena in the ferromagnetic semiconductor (Ga,Mn)As and single crystalline Fe/GaAs (001) hybrid structures. In both systems, spin-orbit interaction is the key ingredient for various intriguing phenomena. -
References
[1] Ohno H, Shen A, Matsukura F, et al. (Ga,Mn)As: A new diluted magnetic semiconductor based on GaAs. Appl Phys Lett, 1996, 69, 363 doi: 10.1063/1.118061[2] Dietl T, Ohno H, Matsukura F, et al. Zener model description in ferromagnetism in zinc-blende magnetic semiconductors. Sience, 2000, 287, 1019 doi: 10.1126/science.287.5455.1019[3] Diet T, Ohno H, Matsukura F. Hole-mediated ferromagnetism in tetrahedrally coordinated semiconductors. Phys Rev B, 2001, 63, 195205 doi: 10.1103/PhysRevB.63.195205[4] Matsukura F, Ohno H. Chapter 19 Molecular beam epitaxy of III–V semiconductors in molecular beam epitaxy from research to mass production (Henini M, Ed.). Amsterdam: Elsevier, 2013[5] Dietl T, Ohno H. Dilute ferromagnetic semiconductors: Physics and spintronic structures. Rev Mod Phys, 2014, 86, 187 doi: 10.1103/RevModPhys.86.187[6] Jungwirth T, Wunderlich J, Novák, V, et al. Spin-dependent phenomena and device concepts explored in (Ga,Mn)As. Rev Mod Phys, 2014, 86, 855 doi: 10.1103/RevModPhys.86.855[7] Schneider J, Kaufmann, Y, Wilkening W, et al. Electronic structure of neutral manganese acceptor in gallium arsenide. Phys Rev Lett, 1987, 59, 240 doi: 10.1103/PhysRevLett.59.240[8] Szczytko J, Teardowski J, Świątek K, et al. Mn impurity in Ga1–xMnxAs epilayers. Phys Rev B, 1999, 60, 8304 doi: 10.1103/PhysRevB.60.8304[9] Yu K M, Walukiewicz W, Wojtowicz T, et al. Effect of the location of Mn sites in ferromagnetic Ga1–xMnxAs on its Curie temperature. Phys Rev B, 2002, 65, 201303 doi: 10.1103/PhysRevB.65.201303[10] Blinowski J, Kacman P. Spin interactions of interstitial Mn ions in ferromagnetic GaMnAs. Phys Rev B, 2003, 67, 121204(R) doi: 10.1103/PhysRevB.67.121204[11] Wojtowicz T, Furdyna J K, Liu X, et al. Electronic effects determining the formation of ferromagnetic III1–xMnxV alloys during epitaxial growth. Physica E, 2004, 25, 171 doi: 10.1016/j.physe.2004.06.014[12] Edmonds K W, Bogusławski P, Wang K Y, et al. Mn interstitial diffusion in (Ga,Mn)As. Phys Rev Lett, 2004, 92, 037201 doi: 10.1103/PhysRevLett.92.037201[13] Souma S, Chen L, Oszwałdowski R. Fermi level position, Coulomb gap, and Dresselhaus splitting in (Ga,Mn)As. Sci Rep, 2016, 6, 27266 doi: 10.1038/srep27266[14] Fid K F, Sheu B L, Maksimov O, et al. Nanoengineered Curie temperature in laterally patterned ferromagnetic semiconductor heterostructures. Appl Phys Lett, 2005, 86, 152505 doi: 10.1063/1.1900938[15] Chen L, Yan X, Yang F, et al. Enhancing the Curie temperature of ferromagnetic semiconductor (Ga,Mn)As to 200 K via nanostructure engineering. Nano Lett, 2011, 11, 2584 doi: 10.1021/nl201187m[16] Shen A, Ohno H, Matsukura F, et al. Epitaxy of (Ga,Mn)As, a new diluted magnetic semiconductor based on GaAs. J Cryst Growth, 1997, 175/176, 1069 doi: 10.1016/S0022-0248(96)00967-0[17] Jungwirth T, Niu Q, MacDonald A H. Anomalous Hall effect in ferromagnetic semiconductors. Phys Rev Lett, 2002, 88, 207208 doi: 10.1103/PhysRevLett.88.207208[18] Baxter D V, Ruzmetov D, Scherschligt J, et al. Anisotropic magnetoresistance in Ga1–xMnxAs. Phys Rev B, 2002, 65, 212407 doi: 10.1103/PhysRevB.65.212407[19] Tang H X, Kawakami R K, Awschalom D D, et al. Giant planar Hall effect in epitaxial (Ga,Mn)As devices. Phys Rev Lett, 2003, 90, 107201 doi: 10.1103/PhysRevLett.90.107201[20] Pappert K, Hümpfner S, Wenisch J, et al. Transport characterization of the magnetic anisotropy of (Ga,Mn)As. Appl Phys Lett, 2007, 90, 062109 doi: 10.1063/1.2437075[21] Yamada T, Chiba D, Matsukura F, et al. Magnetic anisotropy in (Ga,Mn)As probed by magnetotransport measurements. Phys Status Solidi C, 2006, 3, 4086 doi: 10.1002/(ISSN)1610-1642[22] Abolfath M, Jungwirth T, Brum J, et al. Theory of magnetic anisotropy in III1–xMnxV ferromagets. J Magn Magn Mater, 2008, 320, 1190 doi: 10.1016/j.jmmm.2007.12.019[23] Birowska M, Śliwa C, Majewski J A, et al. Origin of bulk uniaxial anisotropy in zinc-blende dilute magnetic semiconductors. Phys Rev Lett, 2012, 108, 237203 doi: 10.1103/PhysRevLett.108.237203[24] Zemen J, Kučera J, Olejník K, et al. Magnetocrystalline anisotropies in (Ga,Mn)As: Systematic theoretical study and comparison with experiment. Phys Rev B, 2009, 80, 155203 doi: 10.1103/PhysRevB.80.155203[25] Stefanowicz W, Śliwa C, Alekshkevych P, et al. Magnetic anisotropy of epitaxial (Ga,Mn)As on (113)A GaAs. Phys Rev B, 2010, 81, 155203 doi: 10.1103/PhysRevB.81.155203[26] Sawicki M, Poselkov O, Sliwa C, et al. Cubic anisotropy in (Ga,Mn)As layers: Experiment and theory. Phys Rev B, 2018, 97, 184403 doi: 10.1103/PhysRevB.97.184403[27] Oiwa A, Katsumoto S, Endo A, et al. Nonmetal-metal-nonmetal transition and large negative magnetoresistance in (Ga,Mn)As/GaAs. Solid State Commun, 1997, 103, 209 doi: 10.1016/S0038-1098(97)00178-6[28] Dietl T. Interplay between carrier localization and magnetism in diluted magnetic and ferromagnetic semiconductors. J Phys Soc Jpn, 2008, 77, 031005 doi: 10.1143/JPSJ.77.031005[29] Sawicki M, Chiba D, Korbecka A. Experimental probing of the interplay between ferromagnetism and localization in (Ga,Mn)As. Nat Phys, 2009, 6, 22 doi: 10.1038/nphys1455[30] Chen L, Matsukura F, Ohno, H. Electric-field modulation of damping constant in a ferromagnetic semiconductor (Ga,Mn)As. Phys Rev Lett, 2015, 115, 057204 doi: 10.1103/PhysRevLett.115.057204[31] Chiba D, Matsukura F, Ohno H. Electric-field control of ferromagnetism in (Ga,Mn)As. Appl Phys Lett, 2006, 89, 162505 doi: 10.1063/1.2362971[32] Chiba D, Sawicki M, Nishitani Y, et al. Magnetization vector manipulation by electric fields. Nature, 2008, 455, 515 doi: 10.1038/nature07318[33] Chiba D, Werpachowska, A, Endo M, et al. Anomalous Hall effect in field-effect structures of (Ga,Mn)As. Phys Rev Lett, 2010, 104, 106601 doi: 10.1103/PhysRevLett.104.106601[34] Matsukura F, Tokura Y, Ohno H. Control of magnetism by electric fields. Nat Nanotechnol, 2015, 10, 209 doi: 10.1038/nnano.2015.22[35] Liu X, Furdyna J K. Ferromagnetic resonance in Ga1–xMnxAs dilute magnetic semiconductors. J Phys: Condens Matter, 2006, 18, R245 doi: 10.1088/0953-8984/18/13/R02[36] Gilbert T L. A phenomenological theory of damping in ferromagnetic materials. IEEE Trans Magn, 2004, 40, 3443 doi: 10.1109/TMAG.2004.836740[37] Chen L, Matsukura F, Ohno H. Direct-current voltages in (Ga,Mn)As structures induced by ferromagnetic resonance. Nat Commun, 2013, 4, 2055 doi: 10.1038/ncomms3055[38] Suhl H. Ferromagnetic resonance in nickel ferrite between one and two kilomegacycles. Phys Rev, 1955, 97, 555 doi: 10.1103/PhysRev.97.555.2[39] Mizukami S, Ando Y, Miyazaki, T. The study on ferromagnetic resonance linewidth for NM/80NiFe/NM (NM = Cu, Ta, Pd and Pt) films. Jpn J Appl Phys, 2001, 40, 580 doi: 10.1143/JJAP.40.580[40] Arias R, Mills D L. Extrinsic contributions to the ferromagnetic resonance response of ultrathin films. Phys Rev B, 1999, 60, 7395 doi: 10.1103/PhysRevB.60.7395[41] Lindner J, Barsukov, I, Raeder C, et al. Two-magnon damping in thin films in case of canted magnetization: Theory versus experiment. Phys Rev B, 2009, 80, 224421 doi: 10.1103/PhysRevB.80.224421[42] Okada A, Kanai S, Yamanouchi M, et al. Electric-field effects on magnetic anisotropy and damping constant in Ta/CoFeB/MgO investigated by ferromagnetic resonance. Appl Phys Lett, 2014, 105, 052415 doi: 10.1063/1.4892824[43] Juretschke H J. Electromagnetic theory of dc effects in ferromagnetic resonance. J Appl Phys, 1960, 31, 1401 doi: 10.1063/1.1735851[44] Fang D, Kurebayashi H, Wunderlich J, et al. Spin-orbit-driven ferromagnetic resonance. Nat Nanotechnol, 2011, 6, 413 doi: 10.1038/nnano.2011.68[45] Mizukami S, Ando Y, Miyazaki T. Effect of spin diffusion on Gilbert damping for a very thin permalloy layer in Cu/permalloy/ Cu/Pt films. Phys Rev B, 2002, 66, 104413 doi: 10.1103/PhysRevB.66.104413[46] Tserkovnyak Y, Brataas A, Bauer G E W. Enhanced Gilbert damping in thin ferromagnetic films. Phys Rev Lett, 2002, 88, 117601 doi: 10.1103/PhysRevLett.88.117601[47] Saitoh E, Ueda M, Miyajima, H, et al. Conversion of spin current into charge current at room temperature: Inverse spin-Hall effect. Appl Phys Lett, 2006, 88, 182509 doi: 10.1063/1.2199473[48] Chen L, Ikeda S, Matsukura F, et al. DC voltages in Py and Py/Pt under ferromagnetic resonance. Appl Phys Express, 2014, 7, 013002 doi: 10.7567/APEX.7.013002[49] Nakayama H, Chen L, Chang H W, et al. Inverse spin Hall effect in Pt/(Ga,Mn)As. Appl Phys Lett, 2015, 106, 222405 doi: 10.1063/1.4922197[50] Isogami S, Tsunoda M. Enhanced inverse spin-Hall voltage in (001) oriented Fe4N/Pt polycrystalline films without contribution of planar-Hall effect. Jpn J Appl Phys, 2016, 55, 043001 doi: 10.7567/JJAP.55.043001[51] Chernyshov A, Overby M, Liu X, et al. Evidence for reversible control of magnetization in a ferromagnetic material by means of spin-orbit magnetic field. Nat Phys, 2009, 5, 656 doi: 10.1038/nphys1362[52] Endo M, Matsukura F, Ohno H. Current induced effective magnetic field and magnetization reversal in uniaxial anisotropy (Ga,Mn)As. Appl Phys Lett, 2010, 97, 222501 doi: 10.1063/1.3520514[53] Moser J, Matos-Abiague A, Schuh D, et al. Tunneling anisotropic magnetoresistance and spin-orbit coupling in Fe/GaAs/Au tunnel junctions. Phys Rev Lett, 2007, 99, 056601 doi: 10.1103/PhysRevLett.99.056601[54] Gmitra M, Matos-Abiague A, Draxl C, et al. Magnetic control of spin-orbit fields: A first-principles study of Fe/GaAs junctions. Phys Rev Lett, 2013, 111, 036603 doi: 10.1103/PhysRevLett.111.036603[55] Žutić I, Fabian J, Das Sarma S. Spintronics: Fundamentals and applications. Rev Mod Phys, 2004, 76, 323 doi: 10.1103/RevModPhys.76.323[56] Zhu H J, Ramsteiner M, Kostial H, et al. Room-temperature spin injection from Fe into GaAs. Phys Rev Lett, 2001, 87, 016601 doi: 10.1103/PhysRevLett.87.016601[57] Lou X, Adelmann C, Crooker S A, et al. Electrical detection of spin transport in lateral ferromagnet-semiconductor devices. Nat Phys, 2007, 3, 197 doi: 10.1038/nphys543[58] Chen L, Decker M, Kronseder M, et al. Robust spin-orbit torque and spin-galvanic effect at the Fe/GaAs(001) interface at room temperature. Nat Commun, 2016, 7, 13802 doi: 10.1038/ncomms13802[59] Fabian J, Matos-Abiague A, Ertler C, et al. Semiconductor spintronics. Acta Physics Slovaca, 2007, 57, 565[60] Sánchez J C R, Vila L, Desfonds G, et al. Spin-to-charge conversion using Rashba coupling at the interface between non-magnetic materials. Nat Commun, 2013, 4, 2944 doi: 10.1038/ncomms3944[61] Lesne E, Fu Y, Oyarzun S, et al. Highly efficient and tunable spin-to-charge conversion through Rashba coupling at oxide interfaces. Nat Mater, 2016, 15, 1261 doi: 10.1038/nmat4726[62] Chen L, Gmitra M, Vogel M, et al. Electric-field control of interfacial spin-orbit fields. Nat Elect, 2018, 1, 350 doi: 10.1038/s41928-018-0085-1[63] Liu H, Lim W L, Urazhdin. Control of current-induced spin-orbit effects in a ferromagnetic heterostructure by electric field. Phys Rev B, 2014, 89, 220409(R) doi: 10.1103/PhysRevB.89.220409[64] Hupfauer T, Matos-Abiague A, Gmitra M, et al. Emergence of spin-orbit fields in magnetotransport of quasi-two-dimensional iron on gallium arsenide. Nat Commun, 2015, 6, 7374 doi: 10.1038/ncomms8374[65] Buchner M, Högl P, Putz S, et al. Anisotropic polar magneto-optic Kerr effect of ultrathin Fe/GaAs (001) layers due to interfacial spin-orbit interaction. Phys Rev Lett, 2016, 117, 157202 doi: 10.1103/PhysRevLett.117.157202[66] Chen L, Mankovsky S, Wimmer S, et al. Emergence of anisotropic Gilbert damping in ultrathin Fe layers on GaAs(001). Nat Phys, 2018, 14, 490 doi: 10.1038/s41567-018-0053-8 -
Proportional views






 DownLoad:
DownLoad: