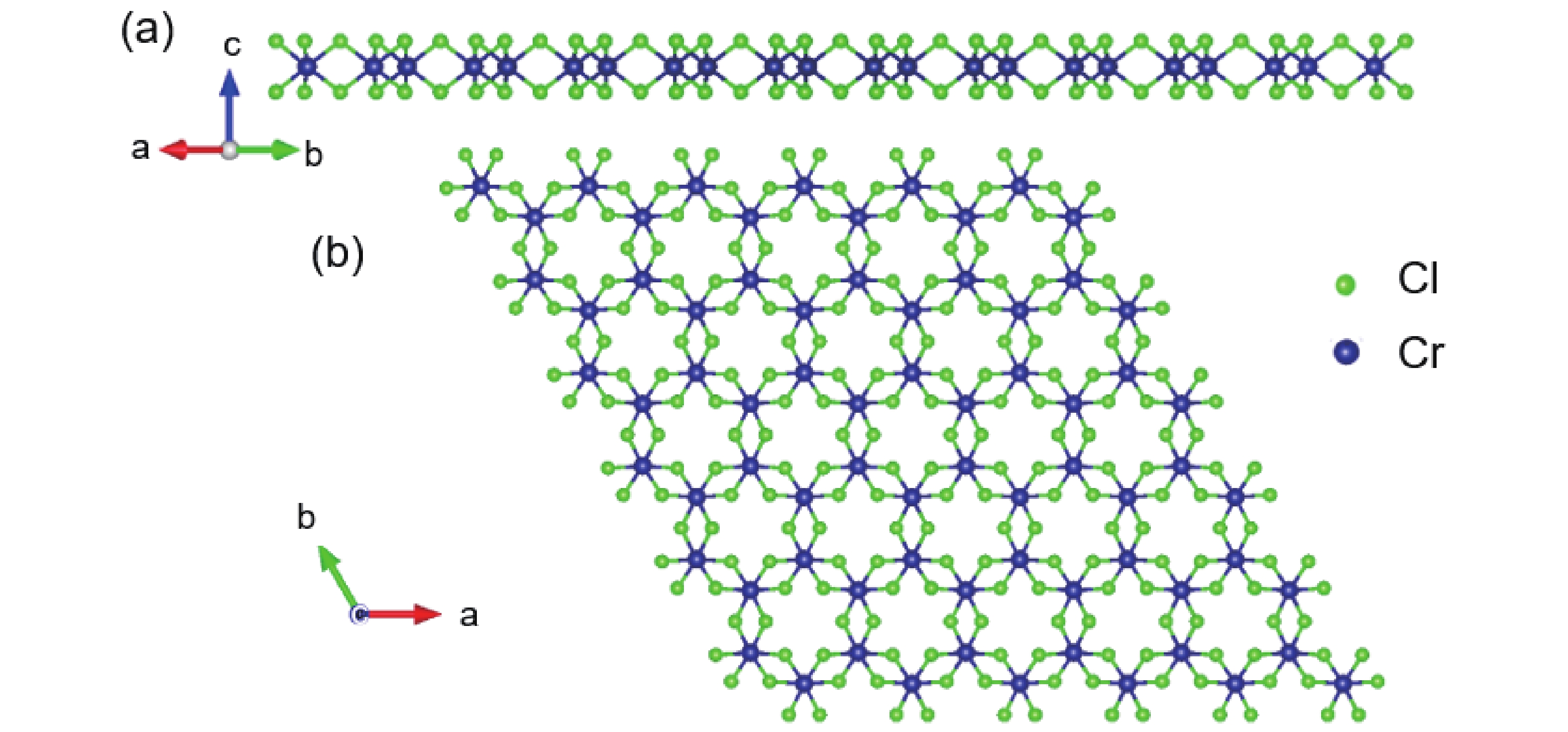
Fig. 1.
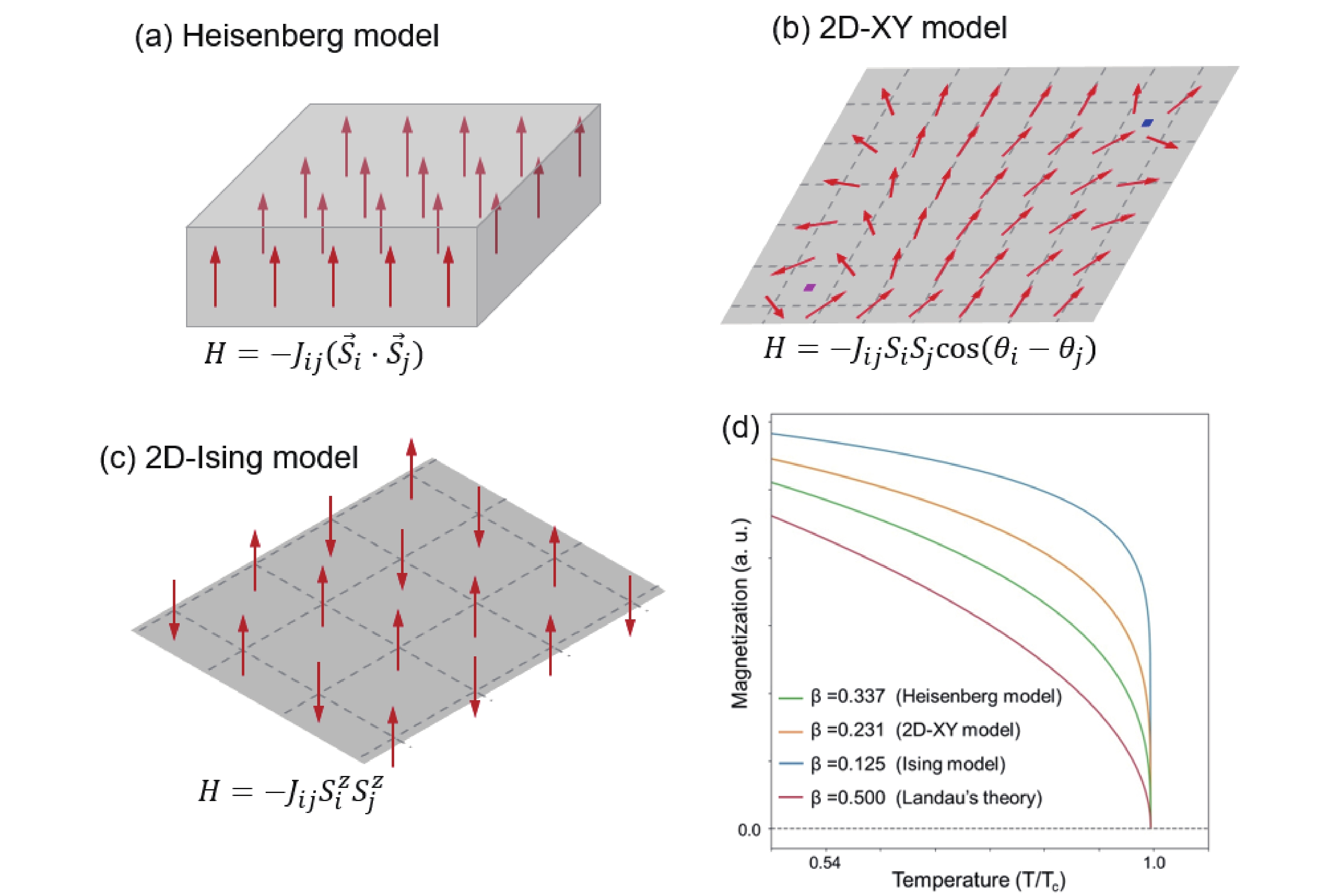
(Color online) (a–c) Schematic diagrams of different mechanisms of ferroelectric phase transition without applied external magnetic field in (a) Heisenberg model, (b) 2D-XY model, and (c) 2D-Ising model. (d) Theoretical comparison of M–T curves around TC under different mechanisms indicated by corresponding critical exponent β.
| Citation: |
Jiesu Wang. Berezinskii-Kosterlitz-Thouless phase transition in a 2D-XY ferromagnetic monolayer[J]. Journal of Semiconductors, 2021, 42(12): 120401. doi: 10.1088/1674-4926/42/12/120401
****
J S Wang, Berezinskii-Kosterlitz-Thouless phase transition in a 2D-XY ferromagnetic monolayer[J]. J. Semicond., 2021, 42(12): 120401. doi: 10.1088/1674-4926/42/12/120401.
|
Berezinskii-Kosterlitz-Thouless phase transition in a 2D-XY ferromagnetic monolayer
DOI: 10.1088/1674-4926/42/12/120401
More Information
-
References
[1] Hohenberg P C. Existence of long-range order in one and two dimensions. Phys Rev, 1967, 158, 383 doi: 10.1103/PhysRev.158.383[2] Mermin N D, Wagner H. Absence of ferromagnetism or antiferromagnetism in one- or two-dimensional isotropic Heisenberg models. Phys Rev Lett, 1966, 17, 1133 doi: 10.1103/PhysRevLett.17.1133[3] Berezinskiǐ V. Destruction of long-range order in one-dimensional and two-dimensional systems possessing a continuous symmetry group. II. Quantum systems. Sov Phys JETP, 1972, 34, 610[4] Kosterlitz J M, Thouless D J. Ordering, metastability and phase transitions in two-dimensional systems. J Phys C, 1973, 6, 1181 doi: 10.1088/0022-3719/6/7/010[5] Kosterlitz J M. The critical properties of the two-dimensional xy model. J Phys C, 1974, 7, 1046 doi: 10.1088/0022-3719/7/6/005[6] Chaikin P M, Lubensky T C. Principles of condensed matter physics. Cambridge, UK: Cambridge University Press, 2020[7] Pires T, Sergio A. Theoretical tools for spin models in magnetic systems. IOP ebooks. Bristol, UK: IOP Publishing, 2021[8] Bedoya-Pinto A, Ji J R, Pandeya A K, et al. Intrinsic 2D-XY ferromagnetism in a van der Waals monolayer. Science, 2021, 374, 616 doi: 10.1126/science.abd5146[9] Huang B, Clark G, Navarro-Moratalla E, et al. Layer-dependent ferromagnetism in a van der Waals crystal down to the monolayer limit. Nature, 2017, 546, 270 doi: 10.1038/nature22391[10] Ghazaryan D, Greenaway M T, Wang Z, et al. Magnon-assisted tunneling in van der Waals heterostructures based on CrBr3. Nat Electron, 2018, 1, 344 doi: 10.1038/s41928-018-0087-z[11] Gong C, Li L, Li Z L, et al. Discovery of intrinsic ferromagnetism in two-dimensional van der Waals crystals. Nature, 2017, 546, 265 doi: 10.1038/nature22060[12] Deng Y J, Yu Y J, Song Y C, et al. Gate-tunable room-temperature ferromagnetism in two-dimensional Fe3GeTe2. Nature, 2018, 563, 94 doi: 10.1038/s41586-018-0626-9[13] Bonilla M, Kolekar S, Ma Y J, et al. Strong room-temperature ferromagnetism in VSe2 monolayers on van der Waals substrates. Nat Nanotechnol, 2018, 13, 289 doi: 10.1038/s41565-018-0063-9[14] Webster L, Yan J A. Strain-tunable magnetic anisotropy in monolayer CrCl3, CrBr3, and CrI3. Phys Rev B, 2018, 98, 144411 doi: 10.1103/PhysRevB.98.144411[15] Stöhr J, Siegman H C. Magnetism: From fundamentals to nanoscale dynamics. Berlin, Germany: Springer, 2006[16] Takei S, Tserkovnyak Y. Superfluid spin transport through easy-plane ferromagnetic insulators. Phys Rev Lett, 2014, 112, 227201 doi: 10.1103/PhysRevLett.112.227201[17] Kim S K, Chung S B. Transport signature of the magnetic Berezinskii-Kosterlitz-Thouless transition. SciPost Phys, 2021, 10, 068[18] Lu X, Fei R, Zhu L, et al. Meron-like topological spin defects in monolayer CrCl3. Nat Commun, 2020, 11, 4724 doi: 10.1038/s41467-020-18573-8[19] Augustin M, Jenkins S, Evans R F L, et al. Properties and dynamics of meron topological spin textures in the two-dimensional magnet CrCl3. Nat Commun, 2021, 12, 185 doi: 10.1038/s41467-020-20497-2 -
Proportional views





 DownLoad:
DownLoad: