| Citation: |
Zhenyao Li, Jia-Min Lai, Jun Zhang. Review of phonons in moiré superlattices[J]. Journal of Semiconductors, 2023, 44(1): 011902. doi: 10.1088/1674-4926/44/1/011902
****
Z Y Li, J M Lai, J Zhang. Review of phonons in moiré superlattices[J]. J. Semicond, 2023, 44(1): 011902. doi: 10.1088/1674-4926/44/1/011902
|
-
Abstract
Moiré patterns in physics are interference fringes produced when a periodic template is stacked on another similar one with different displacement and twist angles. The phonon in two-dimensional (2D) material affected by moiré patterns in the lattice shows various novel physical phenomena, such as frequency shift, different linewidth, and mediation to the superconductivity. This review gives a brief overview of phonons in 2D moiré superlattice. First, we introduce the theory of the moiré phonon modes based on a continuum approach using the elastic theory and discuss the effect of the moiré pattern on phonons in 2D materials such as graphene and MoS2. Then, we discuss the electron–phonon coupling (EPC) modulated by moiré patterns, which can be detected by the spectroscopy methods. Furthermore, the phonon-mediated unconventional superconductivity in 2D moiré superlattice is introduced. The theory of phonon-mediated superconductivity in moiré superlattice sets up a general framework, which promises to predict the response of superconductivity to various perturbations, such as disorder, magnetic field, and electric displacement field. -
References
[1] Encyclopedia Britannica. Chicago: British Encyclopedia Publishing Company, 2022[2] Kobayashi K. Moiré pattern in scanning tunneling microscopy: Mechanism in observation of subsurface nanostructures. Phys Rev B, 1996, 53, 11091 doi: 10.1103/PhysRevB.53.11091[3] N'Diaye A T, Bleikamp S, Feibelman P J, et al. Two-dimensional Ir cluster lattice on a graphene moiré on Ir(111). Phys Rev Lett, 2006, 97, 215501 doi: 10.1103/PhysRevLett.97.215501[4] Liu Y, Weiss N O, Duan X D, et al. Van der Waals heterostructures and devices. Nat Rev Mater, 2016, 1, 16042 doi: 10.1038/natrevmats.2016.42[5] Lopes Dos Santos J M B, Peres N M R, Castro Neto A H. Graphene bilayer with a twist: Electronic structure. Phys Rev Lett, 2007, 99, 256802 doi: 10.1103/PhysRevLett.99.256802[6] Bistritzer R, MacDonald A H. Moire bands in twisted double-layer graphene. Proc Natl Acad Sci USA, 2011, 108, 12233 doi: 10.1073/pnas.1108174108[7] Cao Y, Fatemi V, Fang S A, et al. Unconventional superconductivity in magic-angle graphene superlattices. Nature, 2018, 556, 43 doi: 10.1038/nature26160[8] Huang D, Choi J, Shih C K, et al. Excitons in semiconductor moiré superlattices. Nat Nanotechnol, 2022, 17, 227 doi: 10.1038/s41565-021-01068-y[9] Schmitt D, Bange J P, Bennecke W, et al. Formation of moiré interlayer excitons in space and time. Nature, 2022, 608, 499 doi: 10.1038/s41586-022-04977-7[10] Tran K, Moody G, Wu F C, et al. Evidence for moiré excitons in van der waals heterostructures. Nature, 2019, 567, 71 doi: 10.1038/s41586-019-0975-z[11] Jin C H, Regan E C, Yan A M, et al. Observation of moiré excitons in WSe2/WS2 heterostructure superlattices. Nature, 2019, 567, 76 doi: 10.1038/s41586-019-0976-y[12] Yan J A, Ruan W Y, Chou M Y. Phonon dispersions and vibrational properties of monolayer, bilayer, and trilayer graphene: Density-functional perturbation theory. Phys Rev B, 2008, 77, 125401 doi: 10.1103/PhysRevB.77.125401[13] Nika D L, Balandin A A. Phonons and thermal transport in graphene and graphene-based materials. Rep Prog Phys Phys Soc G B, 2017, 80, 036502 doi: 10.1088/1361-6633/80/3/036502[14] Ribeiro-Palau R, Zhang C J, Watanabe K, et al. Twistable electronics with dynamically rotatable heterostructures. Science, 2018, 361, 690 doi: 10.1126/science.aat6981[15] Choi Y W, Choi H J. Strong electron-phonon coupling, electron-hole asymmetry, and nonadiabaticity in magic-angle twisted bilayer graphene. Phys Rev B, 2018, 98, 241412 doi: 10.1103/PhysRevB.98.241412[16] Jiang J W, Wang B S, Rabczuk T. Acoustic and breathing phonon modes in bilayer graphene with Moiré patterns. Appl Phys Lett, 2012, 101, 023113 doi: 10.1063/1.4735246[17] Cocemasov A I, Nika D L, Balandin A A. Phonons in twisted bilayer graphene. Phys Rev B, 2013, 88, 12 doi: 10.1103/PhysRevB.88.035428[18] Angeli M, Tosatti E, Fabrizio M. Valley jahn-teller effect in twisted bilayer graphene. Phys Rev X, 2019, 9, 041010 doi: 10.1103/PhysRevX.9.041010[19] Lin M L, Tan Q H, Wu J B, et al. Moiré phonons in twisted bilayer MoS2. ACS Nano, 2018, 12, 8770 doi: 10.1021/acsnano.8b05006[20] Koshino M, Son Y W. Moiré phonons in twisted bilayer graphene. Phys Rev B, 2019, 100, 075416 doi: 10.1103/PhysRevB.100.075416[21] Quan J M, Linhart L, Lin M L, et al. Phonon renormalization in reconstructed MoS2 moiré superlattices. Nat Mater, 2021, 20, 1100 doi: 10.1038/s41563-021-00960-1[22] Wu F C, MacDonald A H, Martin I. Theory of phonon-mediated superconductivity in twisted bilayer graphene. Phys Rev Lett, 2018, 121, 257001 doi: 10.1103/PhysRevLett.121.257001[23] Wang T G, Yuan N F Q, Fu L. Moiré surface states and enhanced superconductivity in topological insulators. Phys Rev X, 2021, 11, 021024 doi: 10.1103/PhysRevX.11.021024[24] Choi Y W, Choi H J. Dichotomy of electron-phonon coupling in graphene moiré flat bands. Phys Rev Lett, 2021, 127, 167001 doi: 10.1103/PhysRevLett.127.167001[25] Han S, Nie X H, Gu S Z, et al. Twist-angle-dependent thermal conduction in single-crystalline bilayer graphene. Appl Phys Lett, 2021, 118, 193104 doi: 10.1063/5.0045386[26] Mahapatra P S, Ghawri B, Garg M, et al. Misorientation-controlled cross-plane thermoelectricity in twisted bilayer graphene. Phys Rev Lett, 2020, 125, 226802 doi: 10.1103/PhysRevLett.125.226802[27] Wiki. Moiré pattern, 2022, https://en.wikipedia.org/wiki/Moir%C3%A9_pattern#Materials_science_and_condensed_matter_physics[28] Jung J, Raoux A, Qiao Z H, et al. Ab initiotheory of moiré superlattice bands in layered two-dimensional materials. Phys Rev B, 2014, 89, 205414 doi: 10.1103/PhysRevB.89.205414[29] San-Jose P, Gutiérrez-Rubio A, Sturla M, et al. Spontaneous strains and gap in graphene on boron nitride. Phys Rev B, 2014, 90, 075428 doi: 10.1103/PhysRevB.90.075428[30] Koshino M, Nam N N T. Effective continuum model for relaxed twisted bilayer graphene and moiré electron-phonon interaction. Phys Rev B, 2020, 101, 195425 doi: 10.1103/PhysRevB.101.195425[31] Lai J M, Farooq M U, Sun Y J, et al. Multiphonon process in Mn-doped ZnO nanowires. Nano Lett, 2022, 22, 5385 doi: 10.1021/acs.nanolett.2c01428[32] Ishizuka H, Fahimniya A, Guinea F, et al. Purcell-like enhancement of electron-phonon interactions in long-period superlattices: Linear-temperature resistivity and cooling power. Nano Lett, 2021, 21, 7465 doi: 10.1021/acs.nanolett.1c00565[33] Sun Z X, Hu Y H. How magical is magic-angle graphene. Matter, 2020, 2, 1106 doi: 10.1016/j.matt.2020.03.010[34] Tan P H. Signatures of moiré excitons. J Semicond, 2019, 40, 040202 doi: 10.1088/1674-4926/40/4/040202[35] Shinokita K, Miyauchi Y, Watanabe K, et al. Resonant coupling of a moiré exciton to a phonon in a WSe2/MoSe2 heterobilayer. Nano Lett, 2021, 21, 5938 doi: 10.1021/acs.nanolett.1c00733[36] Mahapatra P S, Sarkar K, Krishnamurthy H R, et al. Seebeck coefficient of a single van der waals junction in twisted bilayer graphene. Nano Lett, 2017, 17, 6822 doi: 10.1021/acs.nanolett.7b03097[37] Duan S, Cui Y, Yi W, et al. Enhanced thermoelectric performance in black phosphorene via tunable interlayer twist. Small, 2022, 2204197 doi: 10.1002/smll.202204197[38] Peltonen T J, Ojajärvi R, Heikkilä T T. Mean-field theory for superconductivity in twisted bilayer graphene. Phys Rev B, 2018, 98, 220504 doi: 10.1103/PhysRevB.98.220504[39] Lian B, Wang Z J, Bernevig B A. Twisted bilayer graphene: A phonon-driven superconductor. Phys Rev Lett, 2019, 122, 257002 doi: 10.1103/PhysRevLett.122.257002[40] Cao Y, Fatemi V, Demir A, et al. Correlated insulator behaviour at half-filling in magic-angle graphene superlattices. Nature, 2018, 556, 80 doi: 10.1038/nature26154[41] Xu C K, Balents L. Topological superconductivity in twisted multilayer graphene. Phys Rev Lett, 2018, 121, 087001 doi: 10.1103/PhysRevLett.121.087001[42] Yuan N F Q, Fu L. Model for the metal-insulator transition in graphene superlattices and beyond. Phys Rev B, 2018, 98, 045103 doi: 10.1103/PhysRevB.98.045103[43] Kang J, Vafek O. Symmetry, maximally localized wannier states, and a low-energy model for twisted bilayer graphene narrow bands. Phys Rev X, 2018, 8, 031088 doi: 10.1103/PhysRevX.8.031088[44] Witt N, Pizarro J M, Berges J, et al. Doping fingerprints of spin and lattice fluctuations in moiré superlattice systems. Phys Rev B, 2022, 105, L241109 doi: 10.1103/PhysRevB.105.L241109[45] Sadovskii M V. Antiadiabatic phonons, coulomb pseudopotential, and superconductivity in eliashberg—McMillan theory. Jetp Lett, 2019, 109, 166 doi: 10.1134/S0021364019030044[46] Hao Z Y, Zimmerman A M, Ledwith P, et al. Electric field-tunable superconductivity in alternating-twist magic-angle trilayer graphene. Science, 2021, 371, 1133 doi: 10.1126/science.abg0399[47] Park J M, Cao Y, Watanabe K, et al. Tunable strongly coupled superconductivity in magic-angle twisted trilayer graphene. Nature, 2021, 590, 249 doi: 10.1038/s41586-021-03192-0[48] Cong X, Lin M L, Tan P H. Lattice vibration and Raman scattering of two-dimensional van der Waals heterostructure. J Semicond, 2019, 40, 091001 doi: 10.1088/1674-4926/40/9/091001[49] Zhou Z Q, Cui Y, Tan P H, et al. Optical and electrical properties of two-dimensional anisotropic materials. J Semicond, 2019, 40, 061001 doi: 10.1088/1674-4926/40/6/061001 -
Proportional views





 DownLoad:
DownLoad:



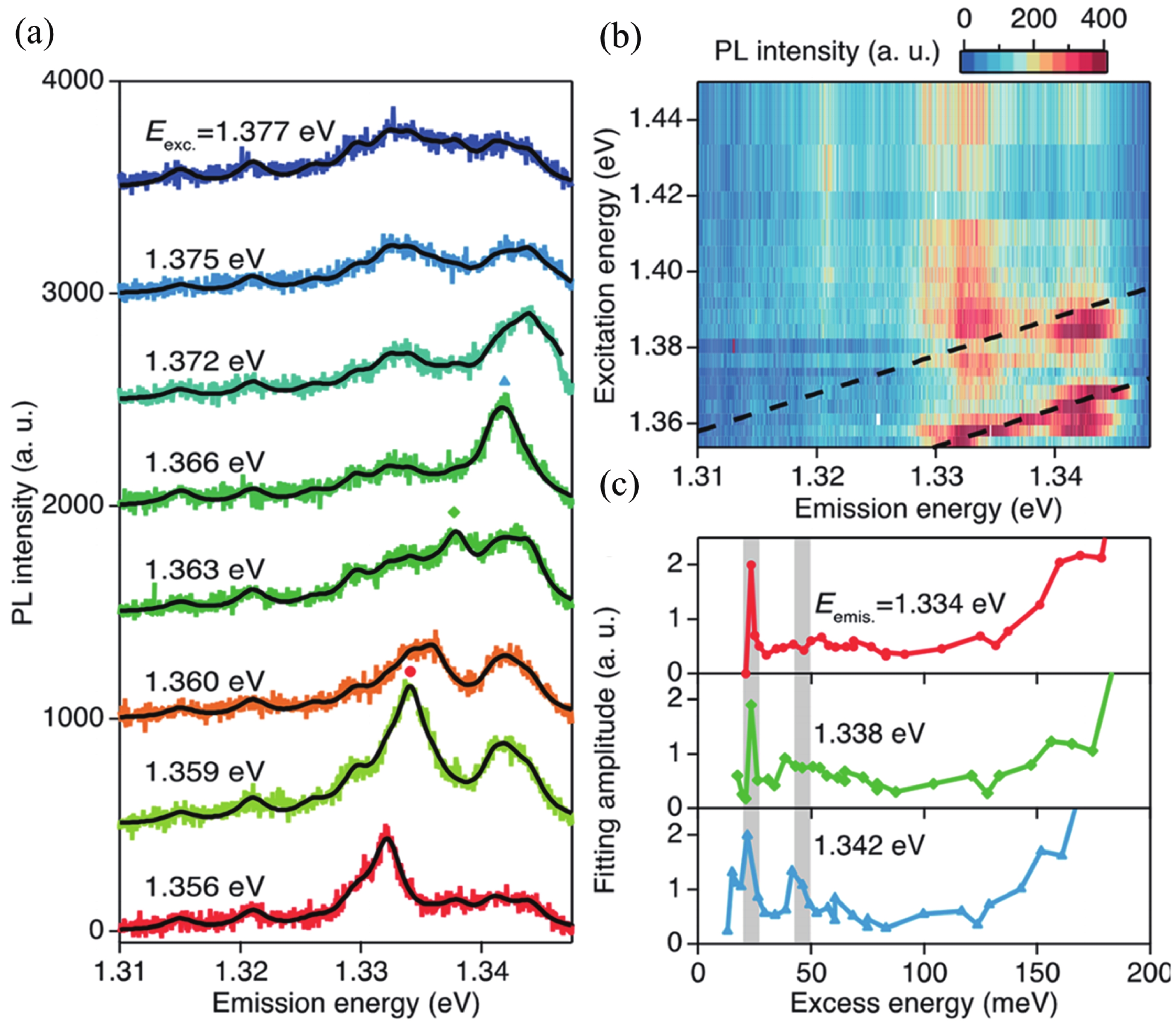
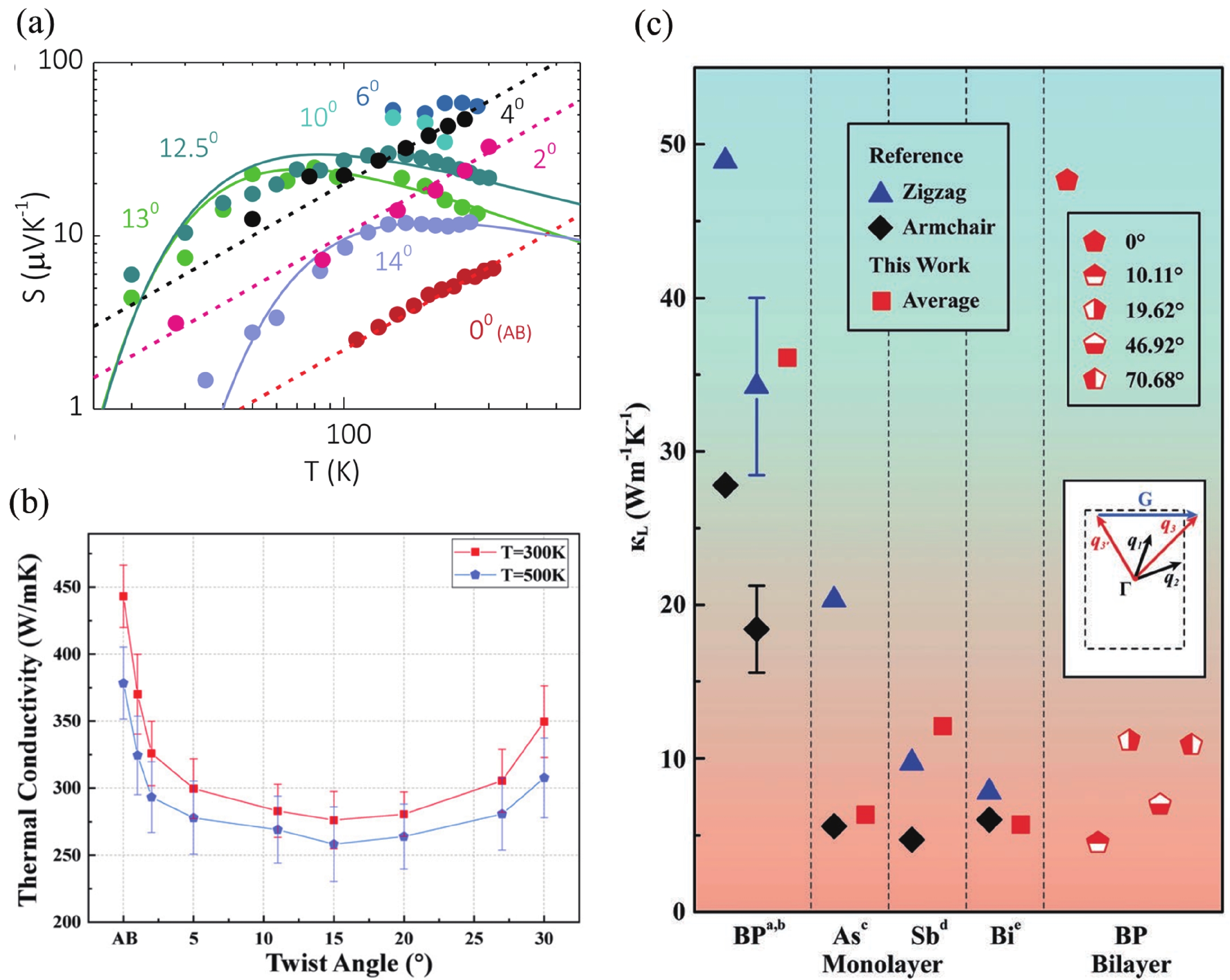












 Zhenyao Li:is now a M.S. student supervised by Prof. Jun Zhang in the State Key Laboratory of Superlattices and Microstructures, Institute of Semiconductors, Chinese Academy of Sciences. He received his bachelor's degree from Nankai University in China. His current research interest focuses on quantum optomechanics
Zhenyao Li:is now a M.S. student supervised by Prof. Jun Zhang in the State Key Laboratory of Superlattices and Microstructures, Institute of Semiconductors, Chinese Academy of Sciences. He received his bachelor's degree from Nankai University in China. His current research interest focuses on quantum optomechanics Jia-Min Lai:is now a Ph.D. student supervised by Prof. Jun Zhang in the State Key Laboratory of Superlattices and Microstructures, Institute of Semiconductors, Chinese Academy of Sciences. She received her bachelor's degree from Northeastern University in China. Her current research interest focuses on electron–phonon coupling in semiconductors
Jia-Min Lai:is now a Ph.D. student supervised by Prof. Jun Zhang in the State Key Laboratory of Superlattices and Microstructures, Institute of Semiconductors, Chinese Academy of Sciences. She received her bachelor's degree from Northeastern University in China. Her current research interest focuses on electron–phonon coupling in semiconductors Jun Zhang:received a bachelor's degree from Inner Mongolia University in China in 2004, and a Ph.D. from the Institute of Semiconductors, Chinese Academy of Sciences in 2010. Then he worked as a postdoctoral fellow at Nanyang Technological University in Singapore from 2010 to 2015 and joined the State Key laboratory of Superlattice for Semiconductors (CAS) as a professor in 2015. His current researches focus on light-matter interactions in semiconductor materials including Raman and Brillouin scattering, and laser cooling in semiconductors
Jun Zhang:received a bachelor's degree from Inner Mongolia University in China in 2004, and a Ph.D. from the Institute of Semiconductors, Chinese Academy of Sciences in 2010. Then he worked as a postdoctoral fellow at Nanyang Technological University in Singapore from 2010 to 2015 and joined the State Key laboratory of Superlattice for Semiconductors (CAS) as a professor in 2015. His current researches focus on light-matter interactions in semiconductor materials including Raman and Brillouin scattering, and laser cooling in semiconductors



