Fig. 1.
(Color online) Analytical fit for the velocity-field relation of double-layer graphene at lattice temperature T = 300 K.
DownLoad:
| Citation: |
Wei Feng. Hydrodynamic simulations of terahertz oscillation in double-layer graphene[J]. Journal of Semiconductors, 2018, 39(12): 122005. doi: 10.1088/1674-4926/39/12/122005
****
F Wei, Hydrodynamic simulations of terahertz oscillation in double-layer graphene[J]. J. Semicond., 2018, 39(12): 122005. doi: 10.1088/1674-4926/39/12/122005.
|
Hydrodynamic simulations of terahertz oscillation in double-layer graphene
DOI: 10.1088/1674-4926/39/12/122005
More Information
-
Abstract
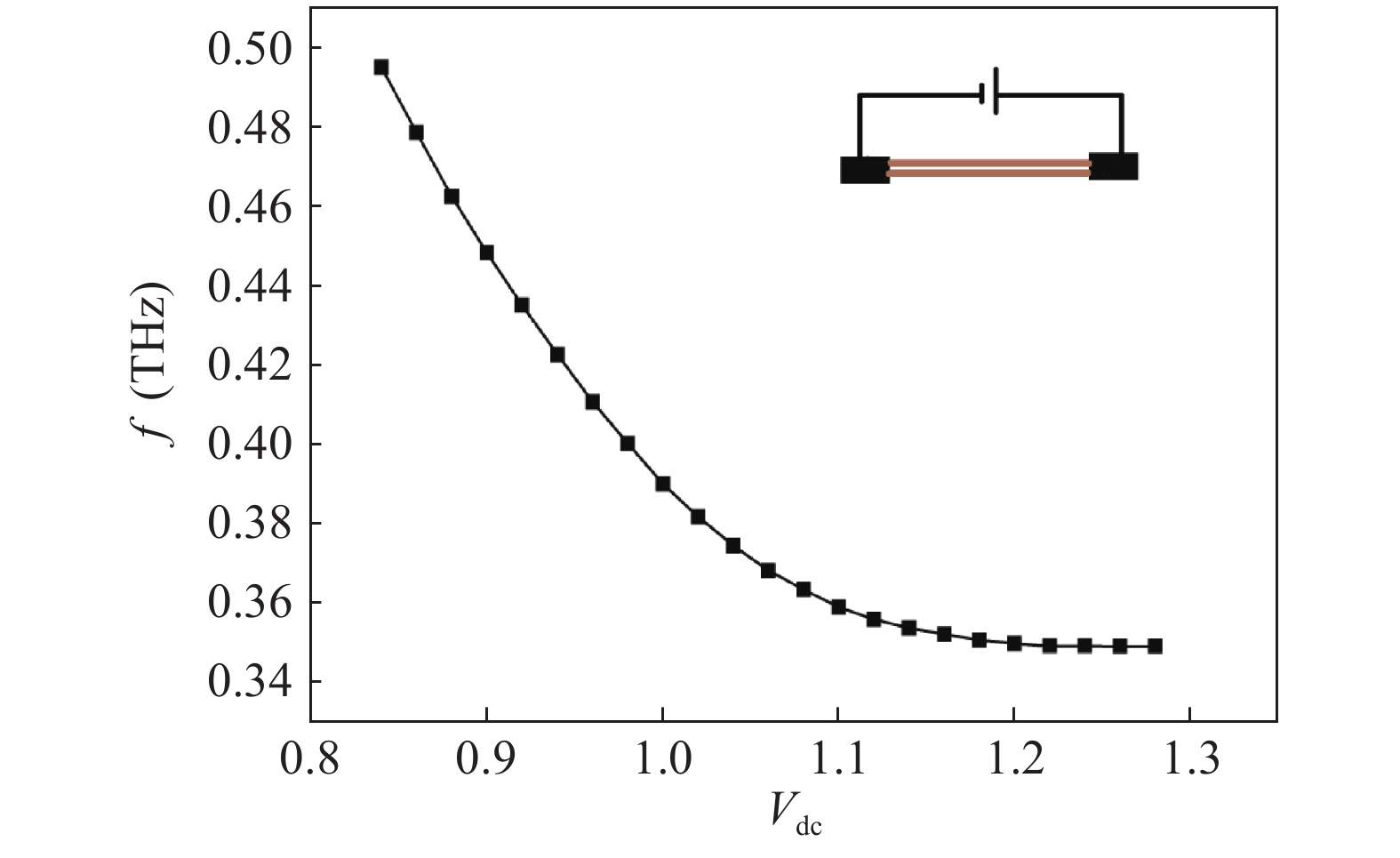
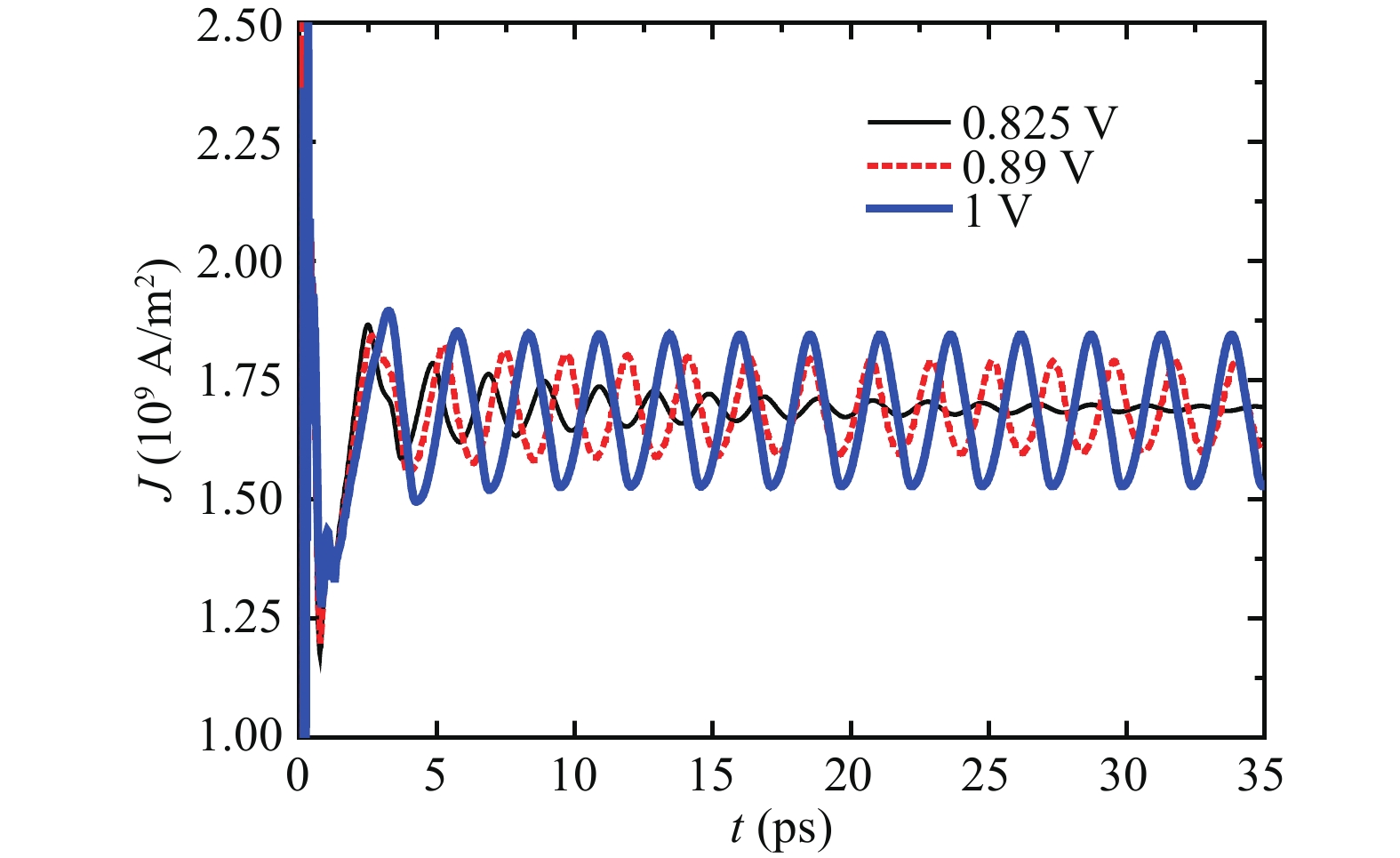
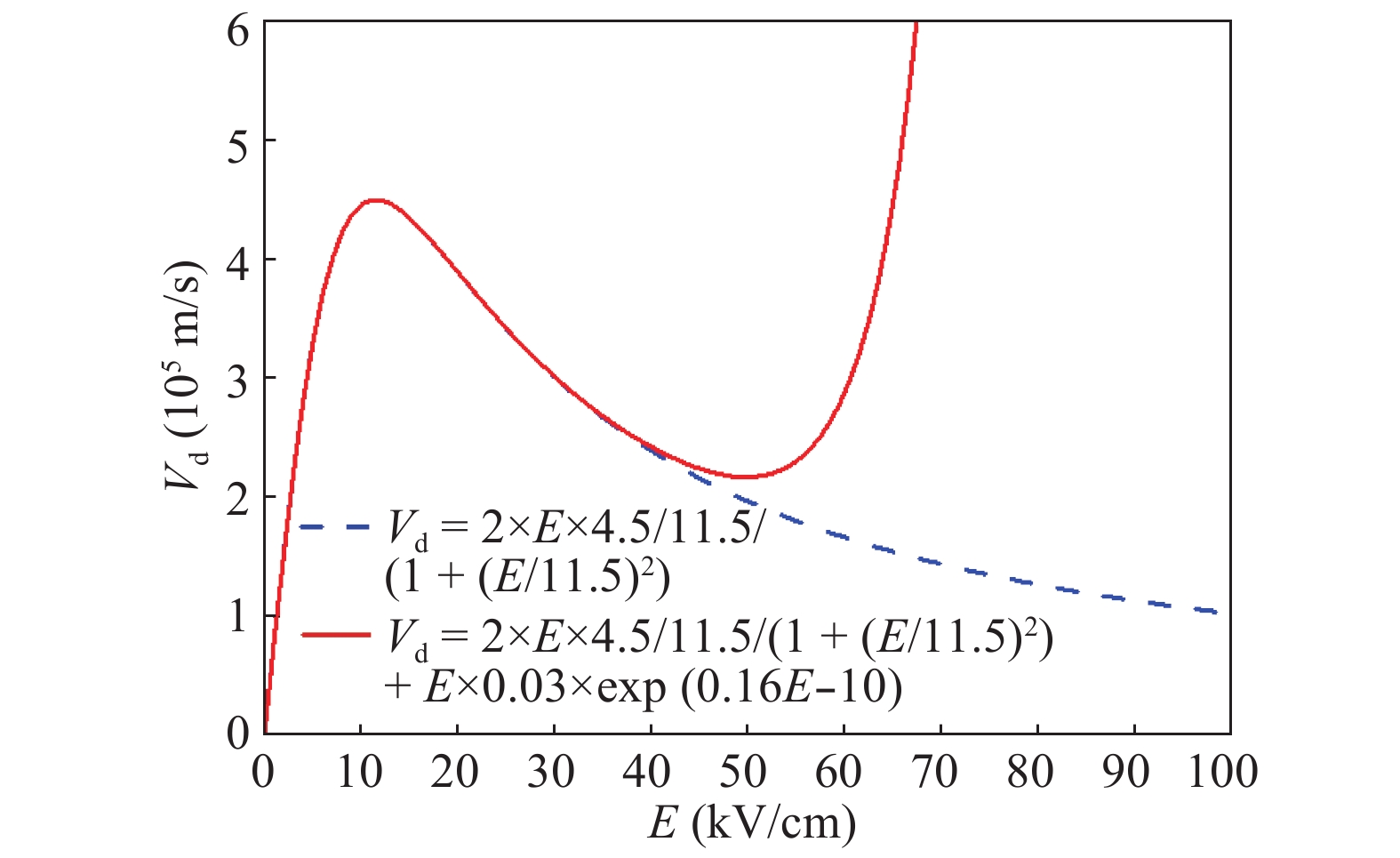
We have theoretically studied current self-oscillations in double-layer graphene n+nn+ diodes driven by dc bias with the help of a time-dependent hydrodynamic model. The current self-oscillation results from resonant tunneling in the double-layer graphene structure. A detailed investigation of the dependence of the current self-oscillations on the applied bias has been carried out. The frequencies of current self-oscillations are in the terahertz (THz) region. The double-layer graphene n+nn+ device studied here may be presented as a THz source at room temperature.-
Keywords:
- terahertz,
- graphene,
- current self-oscillation
-
References
[1] Novoselov KS, Geim A K, Morozov S V, et al. Electric field effect in atomically thin carbon films. Science, 2004, 306: 666 doi: 10.1126/science.1102896[2] Apalkov V M, Chakraborty T. Fractal butterflies in buckled graphenelike materials. Phys Rev B, 2015, 91: 235447 doi: 10.1103/PhysRevB.91.235447[3] Semnani B, Majedi A H, Safavi-Naeini S. Nonlinear quantum optical properties of graphene: the role of chirality and symmetry. Appl Phys Lett, 2015, 85: 115438[4] Zheng Y, Ni G Xn, Toh C T, et al. Graphene field-effect transistors with ferroelectric gating. Phys Rev Lett, 2010, 105: 166602 doi: 10.1103/PhysRevLett.105.166602[5] Ferreira A, Peres N M R, Ribeiro R M, et al. Graphene-based photodetector with two cavities. Phys Rev B, 2012, 85: 115438 doi: 10.1103/PhysRevB.85.115438[6] Bae S, Kim H, Lee Y, et al. Roll-to-roll production of 30-inch graphene films for transparent electrodes. Nat Nanotech, 2010, 5: 574 doi: 10.1038/nnano.2010.132[7] Liu Z, Sanderson M, Zhang C, et al. Nonlinear optical conductivity of bilayer graphene with Rashba spin-orbit interaction in the terahertz regime. J Appl Phys, 2015, 118: 043106 doi: 10.1063/1.4927512[8] Britnell L, Gorbachev R V, Geim A K, et al. Resonant tunnelling and negative differential conductance in graphene transistors. Nat Commun, 2013, 4: 1794 doi: 10.1038/ncomms2817[9] Song Y, Wu H C, Guo Y. Negative differential resistances in graphene double barrier resonant tunneling diodes. Appl Phys Lett, 2013, 102: 093118 doi: 10.1063/1.4794952[10] Nguyen V H, Mazzamuto F, Bournel A, et al. Resonant tunnelling diodes based on graphene/h-BN heterostructure. J Phys D, 2012, 45: 325104 doi: 10.1088/0022-3727/45/32/325104[11] Ferreira G J, Leuenberger M N, Loss D, et al. Low-bias negative differential resistance in graphene nanoribbon superlattices. Phys Rev B, 2011, 84: 125453 doi: 10.1103/PhysRevB.84.125453[12] Ridley B K. X centers in sodium chloride containing calcium. Proc Phys Soc, 1961, 77: 153 doi: 10.1088/0370-1328/77/1/319[13] Feng W, Cao J C. Theoretical study of terahertz current oscillation in GaAs1−xNx. J. Appl. Phys, 2008, 104: 013111 doi: 10.1063/1.2952017[14] Feng W, Cao J C. Nonlinear dynamics in GaAs1−xNx diodes under terahertz radiation. J Appl Phys, 2009, 106: 033708 doi: 10.1063/1.3177345[15] Zhang Z Z, Chang K, Chan K S. Resonant tunneling through double-bended graphene nanoribbons. Appl Phys Lett, 2008, 93: 062106 doi: 10.1063/1.2970957[16] Zhai F. Theory of huge tunneling magnetoresistance in graphene. Phys Rev B, 2008, 77: 113409 doi: 10.1103/PhysRevB.77.113409 -
Proportional views
