| Citation: |
Shihua Chen. Magnetopolaron effects on the optical absorptions in a parabolic quantum dot[J]. Journal of Semiconductors, 2016, 37(9): 092004. doi: 10.1088/1674-4926/37/9/092004
****
S H Chen. Magnetopolaron effects on the optical absorptions in a parabolic quantum dot[J]. J. Semicond., 2016, 37(9): 092004. doi: 10.1088/1674-4926/37/9/092004.
|
Magnetopolaron effects on the optical absorptions in a parabolic quantum dot
DOI: 10.1088/1674-4926/37/9/092004
More Information
-
Abstract
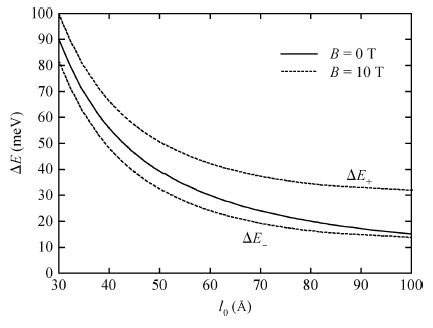
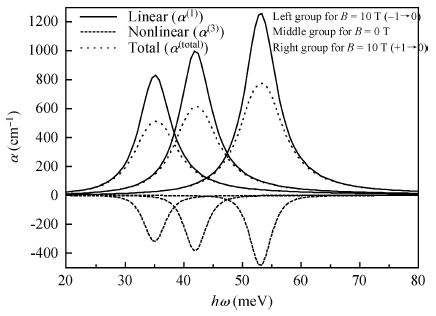
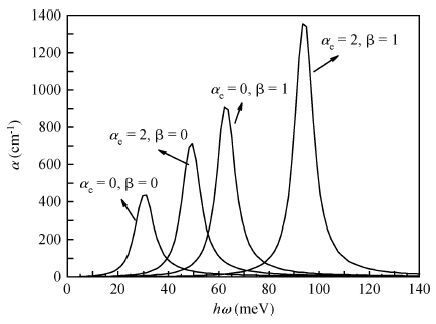
We investigate the influence of magnetic field on the linear and nonlinear optical absorptions in a parabolic quantum dot (QD) through electron-LO-phonon interaction by using the Lee-Low-Pines-Huybrecht variational calculation for all coupling strengths. We apply our calculations to GaAs which is a good candidate in Ⅲ-V group semiconductors. We find that all the absorption spectra are strongly affected by the electron-LO-phonon interaction, the applied magnetic field, and the Coulomb binding potential. Furthermore, due to the Zeeman splitting, the response of all the absorption values in transition (+1→0) and (-1→0) closely depends on the magnetic field increasing.-
Keywords:
- quantum dot,
- magnetopolaron,
- optical absorption
-
References
[1] Bondarenko V, Zhao Y. Resonant photoionization absorption spectra of spherical quantum dots. J Phys Condens Matter, 2003, 15: 1377 doi: 10.1088/0953-8984/15/9/301[2] Yilmaz S, Safak H. Oscillator strengths for the intersubband transitions in a CdS-SiO2 quantum dot with hydrogenic impurity. Physica E, 2007, 36: 40 doi: 10.1016/j.physe.2006.07.040[3] Lu L L, Xie W F, Hassanabadi H. Linear and nonlinear optical absorption coefficients and refractive index changes in a two-electron quantum dot. J Appl Phys, 2011, 109: 063108 doi: 10.1063/1.3560541[4] Sahin M. Photoionization cross section and intersublevel transitions in a one- and two-electron spherical quantum dot with a hydrogenic impurity. Phys Rev B, 2008, 77: 045317 doi: 10.1103/PhysRevB.77.045317[5] Karabulut I, Baskoutas S. Linear and nonlinear optical absorption coefficients and refractive index changes in spherical quantum dots: effects of impurities, electric field, size, and optical intensity. J Appl Phys, 2008, 103: 073512 doi: 10.1063/1.2904860[6] Xie W F. Optical properties of an off-center hydrogenic impurity in a spherical quantum dot with Gaussian potential. Superlatt Microstruct, 2010, 48: 239 doi: 10.1016/j.spmi.2010.04.015[7] Schmitt-Rink S, Miller D A B, Chemla D S. Theory of the linear and nonlinear optical properties of semiconductor microcrystallites. Phys Rev B, 1987, 35: 8113 doi: 10.1103/PhysRevB.35.8113[8] Li Yali, Shan Shuping. Properties of the magnetopolaron in a triangular quantum well. Journal of Semiconductors, 2015, 36(8): 082005 doi: 10.1088/1674-4926/36/8/082005[9] Ma Xinjun, Xiao Boyu, Sun Yong, et al. Effects of magnetic field on the polaron in an asymmetrical Gaussian confinement potential quantum well. Journal of Semiconductors, 2015, 36(10): 102004 doi: 10.1088/1674-4926/36/10/102004[10] Zhang Hairui, Sun Yong. Properties of polaron in a triangular quantum well induced by the Rashba effect. Journal of Semiconductors, 2014, 35(10): 102001 doi: 10.1088/1674-4926/35/10/102001[11] Mukhopadhyay S, Chatterjee A. Suppression of Zeeman splitting in a GaAs quantum dot. Phys Rev B, 1999, 59: R7833 doi: 10.1103/PhysRevB.59.R7833[12] Jacak L, Krasnyj J, Jacak D, et al. Magnetopolaron in a weakly elliptical InAs/GaAs quantum dot. Phys Rev B, 2003, 67: 035303 doi: 10.1103/PhysRevB.67.035303[13] Kandemir B S, Cetin A. Impurity magnetopolaron in a parabolic quantum dot: the squeezed-state variational approach. J Phys Condens Matter, 2005, 17: 667 doi: 10.1088/0953-8984/17/4/009[14] Mukhopadhyay S, Chatterjee A. The ground and the first excited states of an electron in a multidimensional polar semiconductor quantum dot: an all-coupling variational approach. J Phys Condens Matter, 1999, 11: 2071 doi: 10.1088/0953-8984/11/9/005[15] Chen Shihua. The cyclotron resonance of impurity magnetopolarons in two-dimensional quantum dots for all coupling strengths. Physica E, 2011, 43: 1007 doi: 10.1016/j.physe.2010.12.002[16] Xie Wenfang. Optical properties of impurity-bound polaron in a parabolic quantum dot. Superlatt Microstruct, 2011, 50(2): 91 doi: 10.1016/j.spmi.2011.05.002[17] Sahin M. Third-order nonlinear optical properties of a one- and two-electron spherical quantum dot with and without a hydrogenic impurity. J Appl Phys, 2009, 106: 063710 doi: 10.1063/1.3225100[18] Li N, Guo K X, Shao S. Polaron effects on the optical absorptions in cylindrical quantum dots with parabolic potential. Opt Commun, 2012, 285: 2734 doi: 10.1016/j.optcom.2012.01.047[19] Chen Shihua. Properties of the two- and three-dimensional quantum dot qubit. Journal of Semiconductors, 2010, 31: 052001 doi: 10.1088/1674-4926/31/5/052001[20] Chen S H. Optical absorptions of impurity-bound polaron in a GaAs quantum dot with parabolic potential. J Low Temp Phys, 2014, 177: 40 doi: 10.1007/s10909-014-1186-5[21] Peeters F M, Wu X G, Devreese J T. Ground-state energy of a polaron in n dimensions. Phys Rev B, 1986, 33: 3926 doi: 10.1103/PhysRevB.33.3926[22] Vahdani M R K, Rezaei G. Linear and nonlinear optical properties of a hydrogenic donor in lens-shaped quantum dots. Phys Lett A, 2009, 373: 3079 doi: 10.1016/j.physleta.2009.06.042[23] Mukhopadhyay S, Chatterjee A. Polaronic enhancement in the ground-state energy of an electron bound to a Coulomb impurity in a parabolic quantum dot. Phys Rev B, 1997, 55: 9279 doi: 10.1103/PhysRevB.55.9279 -
Proportional views





 DownLoad:
DownLoad: