1. Introduction

Over the last few decades, a lot of research has been done to achieve photonic devices from Ge or Si and its alloys either in bulk form or quantum hetero-structures^{[1, 2]}. However, realization of such photonic devices remained a great challenge due to their indirect band gap and a large lattice mismatch between Si and Ge.

Some recent developments^[3] have been introduced by using alloys of Si and Ge with group IV material like C or α-Sn. The direct growth of GeC thin film on a Ge substrate has been made possible using the molecular beam epitaxy (MBE)^[4]. Recent technology allows a maximum concentration of substitutional C in Ge of only about 3%^[5]. A most important feature of GeC on SiGeSn is that by the application of proper tensile strain, the direct Г-valley conduction band decreases more rapidly than the indirect L-valley band, resulting in an indirect to direct crossover. Under suitable tensile strain, Ge and GeC shows direct band gap type I structure whenever grown on SiGeSn^{[6, 7]}.

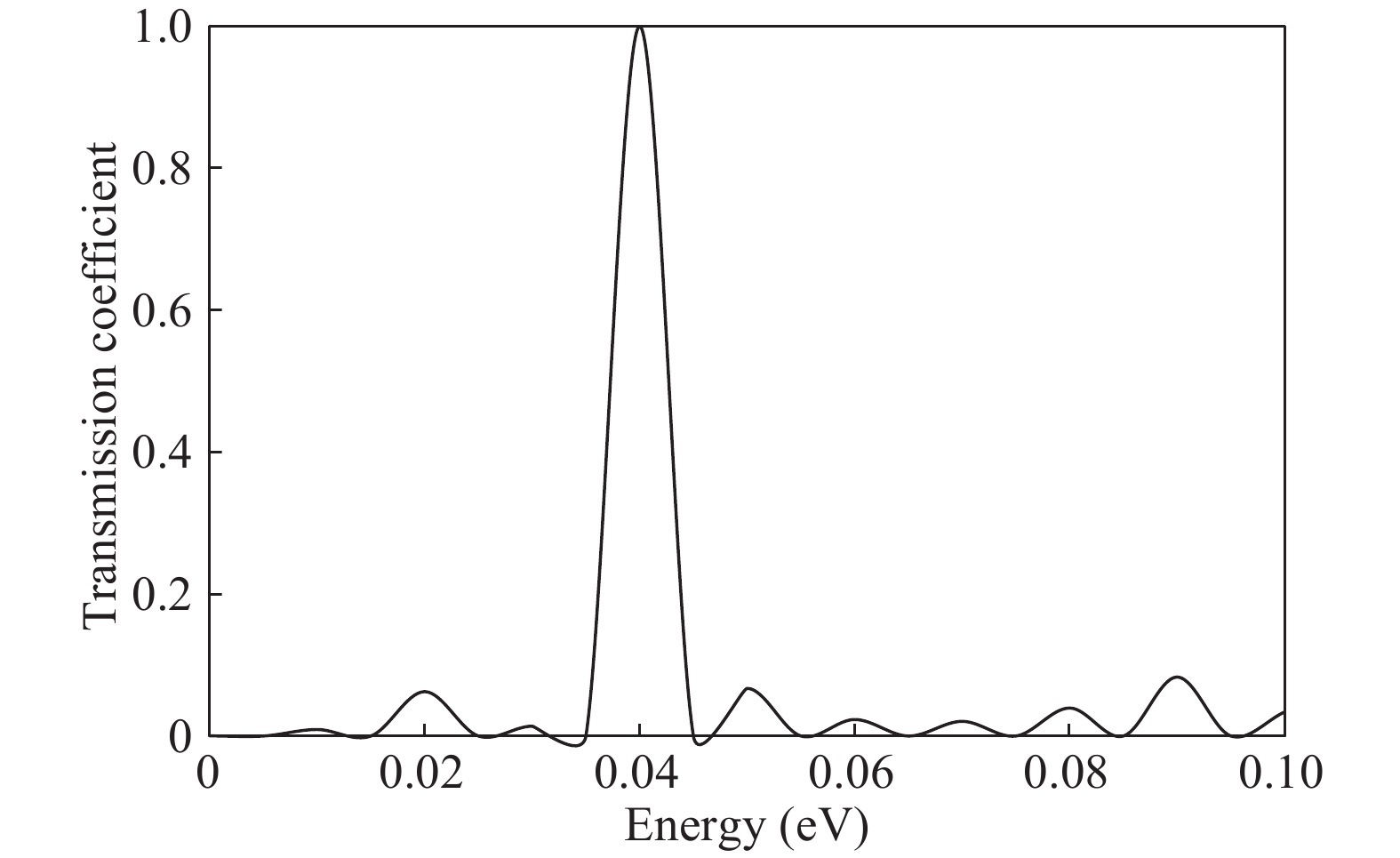
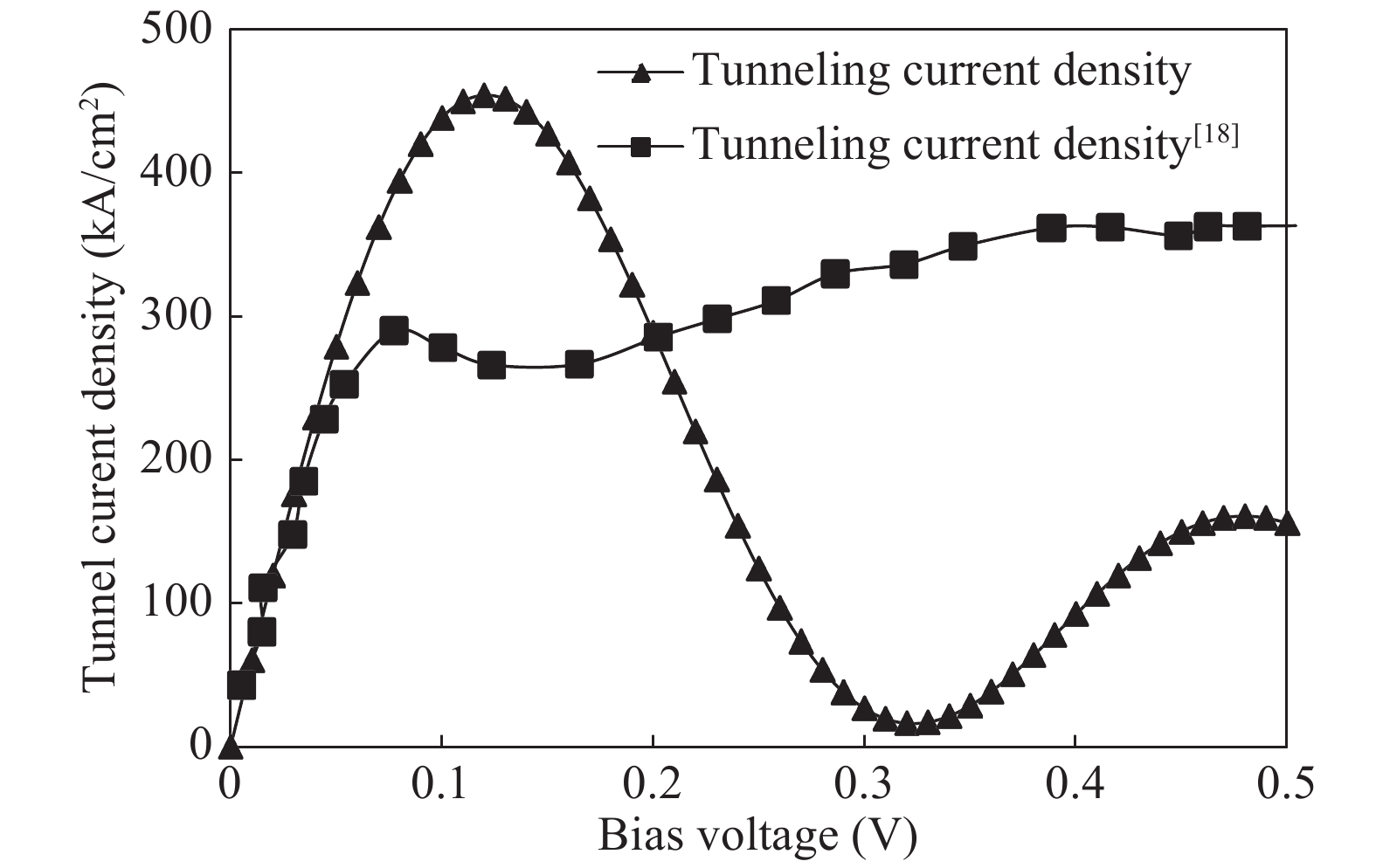
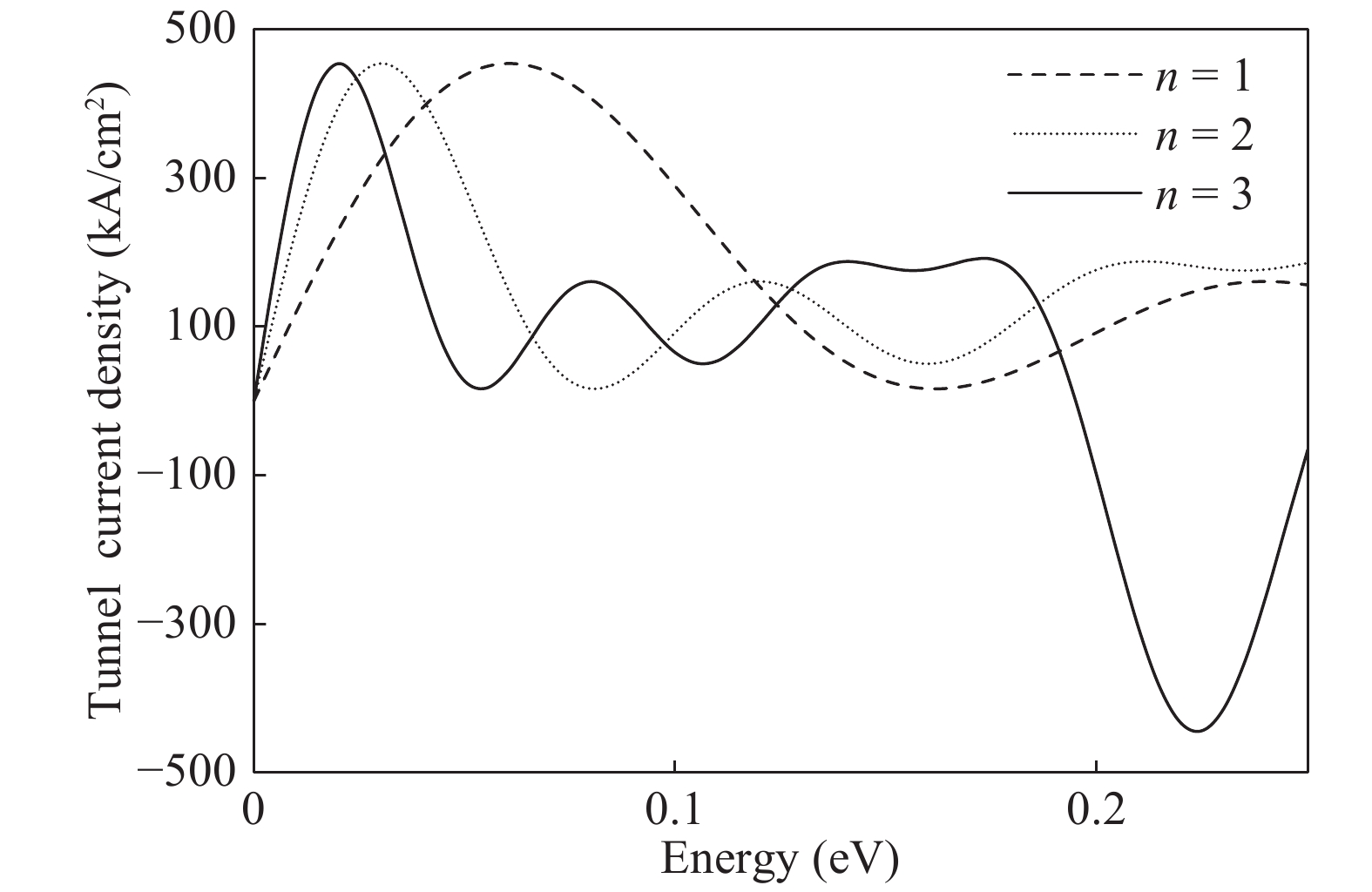
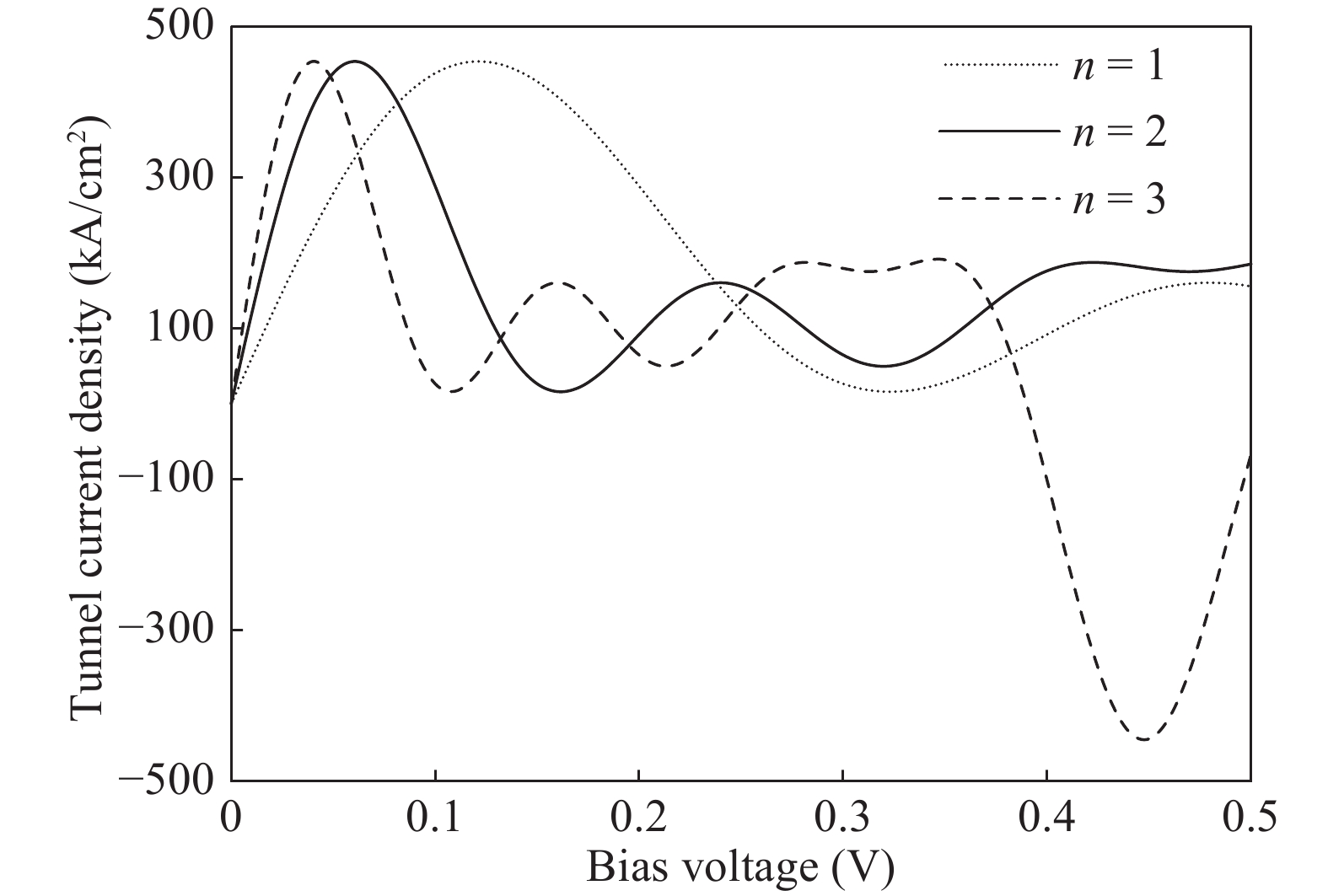
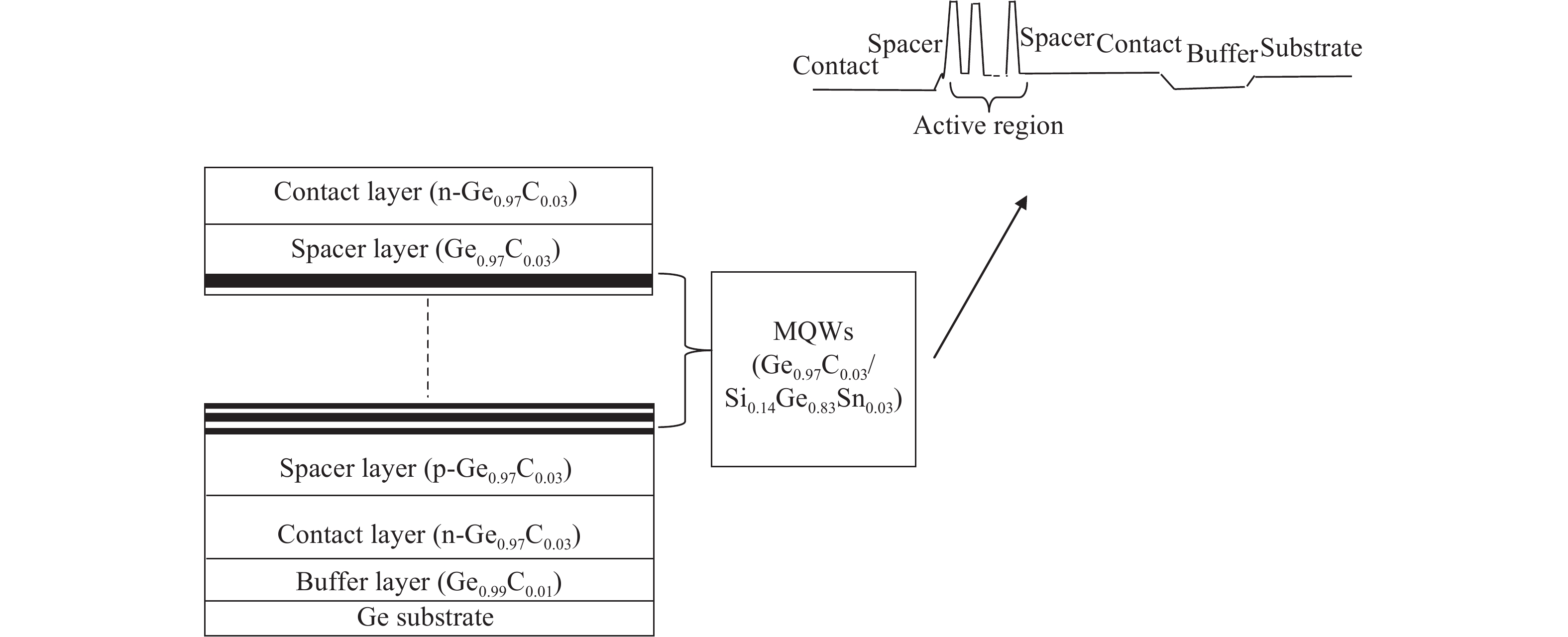
However, with the enormous development of group IV band engineering, GeSn or GeC based multiple quantum wells (MQW) are being used as lasers^[8–11], modulators^{[12, 13]} and quantum well infrared photo detectors (QWIP)^[14]. There has been an immense amount of research attention in the double barrier quantum well (DBQW) resonant tunneling diode (RTD) for nano electronic high speed switching applications due to its high current density. The idea of nanofabrication was popularized on the resonant tunnelling diode by the works of Esaki and Tsu^[15–17]. The direct band gap nature of GeC alloy has encouraged us to study GeC based RTD structure in detail.

After a short introduction in Section 1, the detailed theory related to the calculation of strain-induced band structure is discussed. The tunneling current density in GeC/SiGeSn has been estimated in Section 2. Section 3 gives the comparative study of current density of the proposed structure with the relevant structure of RTD proposed by Wu et al.^[18]. A significant improvement has been noticed in all aspects. This follows the conclusion in Section 4.

2. Theory

2.1 RTD structure and band profile

A schematic of RTD structure is shown in Fig. 1. Ge is chosen as the substrate for the proposed model. A fully strain-relaxed Ge_0.99C_0.01 buffer layer of lattice constant 5.6364 Å is grown on a Ge substrate to act as a “virtual substrate”. An n-type Ge_0.97C_0.03 of lattice constant 5.5946 Å layer is grown on it and it acts as a contact layer. Similarly, Ge_0.97C_0.03 is grown which acts as a spacer layer. The electrical transport process is done by these two consecutive layers. The model is chosen as a direct band gap type I structure. A MQW structure (Si_0.14Ge_0.83Sn_0.03/Ge_0.97C_0.03/Si_0.14Ge_0.83- Sn_0.03) is introduced as an active region. The composition of well and barrier materials are chosen in such a way that the well material produces a negligible strain effect with respect to barrier material. For the proposed model, the band gaps and band offsets are estimated with the help of the relations reported at Menendez et al.^[6]. The lattice constant of Ge_0.97C_0.03 (5.5946 Å) wells is smaller than that of the Ge_0.99C_0.01 buffer layer. This mismatch results in a tensile strain of 0.75% in Ge_0.97C_0.03 wells. On the other hand, Si_0.14Ge_0.83Sn_0.03 (5.6502 Å) barriers have a larger lattice constant than the Ge_0.99C_0.01 buffer layer; mismatch causes a compressive strain of 0.24%.

2.2 Calculation of band structure

In this section, the calculation of band offset, bandgap, lattice constant, strain and other parameters of constituent materials are estimated by a linear interpolation technique where bowing parameters^[11] are included during calculation. The following expressions for the band edges of the strained active layer are obtained as^[6],

$$\begin{split}& {E_{{\rm{v}}\Gamma }}\left( {\rm {activelayer}} \right) = - \frac{{{\Delta _0}\left( {\rm {substrate}} \right)}}{3} - \Delta {E_{{\rm{v}},{\rm{ave}}}}\left( {\rm {substrate}} \right)\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\\& \qquad - \frac{{{\Delta _0}\left( {\rm {activelayer}} \right)}}{6} + \delta E_{\rm{h}}^v + \frac{1}{4}\delta {E_{001}}\\& \qquad + \frac{1}{2}\sqrt {\left( {{{\Big({\Delta _0}({\rm {activelayer}}) + \frac{1}{2}\delta E_{001}'\Big)}^2} + 2{{\left( {\delta E_{001}^{''}} \right)}^2}} \right)},\end{split}$$

(1)

$$\begin{split}{E_{{\rm{C}}\Gamma }}\left( {\rm {activelayer}} \right) =\;& {E_{{\rm{v}}\Gamma }}\left( {\rm {activelayer}} \right)\\& + {E_0}\left( {\rm {activelayer}} \right) + \delta E_{\rm{h}}^{\rm C\Gamma },\qquad \qquad \;\;\;\;\end{split}$$

(2)

$$\begin{split}{E_{{\rm{C}}{\rm L}}}\left( {\rm {activelayer}} \right) =\;& {E_{{\rm{v}}\Gamma }}\left( {\rm {activelayer}} \right)\\& + {E_{{\rm{ind}}}}\left( {\rm {activelayer}} \right) + \delta E_{\rm{h}}^{\rm {CL}}.\qquad \qquad \;\end{split}$$

(3)

All the energies are measured using the valence band edge of the virtual substrate as the reference. Using linear interpolation, the spin-orbit splitting in the alloy is obtained as

$${\Delta _0}\left( {\rm {substrate}} \right) = {\Delta _0}\left( {\rm {activelayer}} \right) - 0.26x + 0.47y,$$

(4)

with ${\Delta _0}\left( {\rm {activelayer}} \right)$ = 0.30 eV,

$$\Delta {E_{{\rm{v}},{\rm{ave}}}}\left({\rm {substrate}} \right) = 0.69y - 0.48x,\qquad$$

(5)

$$\delta E_{001}' = - 2\left( {{b_1} + 2{b_2}} \right)\left[ {\frac{{2{C_{12}}}}{{{C_{11}}}} + 1} \right]{e_{11}},\quad\,\,\,\,$$

(6)

$$\delta E_{001}^{''} = - 2\left( {{b_1} - {b_2}} \right)\left[ {\frac{{2{C_{12}}}}{{{C_{11}}}} + 1} \right]{e_{11}},\quad\,\,\,\,\,\,$$

(7)

$$\delta E_{\rm{h}}^v = 2{a_{\rm{v}}}\left[ {1 - \frac{{{C_{12}}}}{{{C_{11}}}}} \right]{e_{11}},$$

(8)

$$\delta E_{\rm{C}}^{{\rm{C}}\Gamma } = 2{a_{\rm{c}}}\left[ {1 - \frac{{{C_{12}}}}{{{C_{11}}}}} \right]{e_{11}},$$

(9)

$$\delta E_{\rm{C}}^{{\rm{C}}{\rm L}} = 2\left[ {{\Xi _{\rm{d}}} + \frac{1}{3}{\rm E _{\rm{u}}}} \right]\left[ {1 - \frac{{{C_{12}}}}{{{C_{11}}}}} \right]{e_{11}},$$

(10)

where the strain e₁₁ is given by,

$${e_{11}} = \frac{{a\left( {\rm {bufferlayer}} \right) - a({\rm {activelayer}})}}{{a({\rm {activelayer}})}}.$$

(11)

The band offset of the conduction band can be expressed as,

$$E_{{\rm{bandoffset}}}^{{\rm C}\Gamma } = {E_{{\rm{C}}\Gamma }}\left( {\rm {substrate}} \right) - {E_{{\rm{C}}\Gamma }}\left( {\rm {activelayer}} \right).$$

(12)

The band gap energy of the barrier is given by^[11],

$$\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\begin{split}& {E_{{\rm{C}}\Gamma }}\left( {{\rm {Si}}{_{1 - x - y}}{\rm Ge}{_x}{{\rm {Sn}}_y}} \right) = x{E_{\rm{g}}}\left( {\rm {Ge}} \right) + y{E_{\rm{g}}}\left( {\rm {Sn}} \right) \\&\quad + \left( {1 - x - y} \right){E_{\rm{g}}}({\rm {Si}}) - b_\eta ^{\rm {SiGe}}x\left( {1 - x - y} \right)\\ & \quad - b_\eta ^{\rm {SiSn}}y\left( {1 - x - y} \right) - b_\eta ^{\rm {GeSn}}\left( {xy} \right).\end{split}$$

(13)

2.3 Calculation of transmission coefficient

This section is completely based on the work of Mukherjee et al.^[19]. Using Schrödinger-Poisson equation they calculated the transmission coefficient of carriers. In the proposed work, an n–p–n RTD model with GeC/SiGeSn MQW structure is studied. The propagation matrix (P) can be written as^[19]

$$\begin{split}P =& {\left[ {\begin{array}{*{20}{c}}{{{\rm e}^{i{k_1}{L_1}}}} & {{{\rm e}^{ - i{k_1}{L_1}}}}\\{{{\rm e}^{i{k_1}{L_1}}}} & { - {{\rm e}^{ - i{k_1}{L_1}}}}\end{array}} \right]^{ - 1}}\left[ {\begin{array}{*{20}{c}}{Ai{{( - {\xi _2})}_{{L_1}}}} & {Bi{{( - \xi )}_{{L_1}}}}\\{ - \frac{a}{{i{k_1}}}A{i'}{{( - {\xi _2})}_{{L_1}}}} & { - \frac{{{a}}}{{i{k_1}}}B{i'}{{( - {\xi _2})}_{{L_1}}}}\end{array}} \right] \ldots \ldots \\&{\left[ {\begin{array}{*{20}{c}}{Ai{{( - {\xi _{{n} - 1}})}_{{L_{{n} - 1}}}}} & {Bi{{( - {\xi _{{n} - 1}})}_{{L_{{{n}} - 1}}}}}\\{A{i'}{{( - {\xi _{{n} - 1}})}_{{L_{{n} - 1}}}}} & {B{i'}{{( - {\xi _{{n} - 1}})}_{{L_{{n} - 1}}}}}\end{array}} \right]^{ - 1}} \times \left[ {\begin{array}{*{20}{c}}{{{\rm e}^{i{k_{n}}{L_{{n} - 1}}}}} & {{{\rm e}^{ - i{k_{n}}{L_{{n} - 1}}}}}\\{ - \frac{{i{k_{n}}}}{a}{{\rm e}^{i{k_{n}}{L_{{n} - 1}}}}} & {\frac{{i{k_{n}}}}{a}{{\rm e}^{ - i{k_{n}}{L_{{n} - 1}}}}}\end{array}} \right],\end{split}$$

(14)

where Ai and Bi are considered as Airy functions^[20], $L_j$ is the x-axis co-ordinate of the interface between the jth and (j + 1)th region, k_j is the wave vector in the jth region and is given by

$${k_{\rm{j}}} = \sqrt {\frac{{2{m^*}\left( {{E_j} - V} \right)}}{{{\hbar ^2}}}} ,$$

(15)

where $\hbar$ is the reduced Plank constant, ${m^ * }$ is the effective mass, ${E_j}$ is the Eigen energy in the jth region, and V is the potential of the unbiased structure.

Now, ${\xi _{\rm{j}}}$ and $a$ can be expressed as

$${\xi _{\rm{j}}} = {(2{m^ * }qe/{\hbar ^2})^{(1/3)}}(x + {E_{{j}}}/qe),$$

(16)

$$a = {(2{m^ * }q{e}/{\hbar ^2})^{(1/3)}},$$

(17)

where q is the electronic charge, and $e$ is the applied electric field which is kept fixed throughout the analysis.

The transmission coefficient ( $\tau$ ) of the whole MQW structure can be calculated by the following equation^[19],

$$\tau = \frac{1}{{{{\left| {{P_{11}}} \right|}^2}}},$$

(18)

where P₁₁ is the first matrix element of the propagation matrix.

2.4 Tunneling current density

Resonant-tunneling occurs when electrons can tunnel through some resonant states at certain energy levels. Initially there is an absence of applied bias so the band of DBQW is in equilibrium. With the application of the critical value of biased voltage (V_bias) to the two electrode contacts of the structure, the band bends downwards to the right. Electrons can tunnel through the double barriers from the spacer layer to the contact layer resulting in a small current. The injected electrons are characterized by its Fermi level. With the increase of bias voltage, the band is tilted up to a threshold level. The bounded energy level in the QW is aligned with the Fermi energy level (E_F). At a certain point, resonant tunneling occurs. As a result, a sharp current can be achieved. The tunneling current reduces with further increase of biased voltage.

The tunnelling current density ( ${J_{\rm{T}}}$ ) can be expressed as^[18]

$${J_{\rm{T}}} = \frac{{q{m^ * }{K_{\rm{B}}}T}}{{2{\pi ^2}{\hbar ^3}}}\int\limits_0^\infty {\tau ({E_{\rm{x}}}){\rm d}{E_{\rm{x}}}} \ln \left[ {\frac{{1 + \exp { \displaystyle\frac{{{E_{\rm{F}}} - {E_{\rm{x}}}}}{{{K_{\rm{B}}}T}}} }}{{1 + \exp { \displaystyle\frac{{{E_{\rm{F}}} - q{V_{{\rm{bias}}}} - {E_{\rm{x}}}}}{{{K_{\rm{B}}}T}}} }}} \right],$$

(19)

where $\tau ({E_{\rm{x}}})$ is the energy-dependent transmission coefficient.

3. Results and discussion

The values of different required parameters are given in Table 1. In this work the band edges and band offsets in the conduction band for the material Ge_1−zC_z/Si_1−x−yGe_xSn_y are estimated.

The transmission coefficient is estimated and plotted in Fig. 2 as a function of energy for a single well. The well width and the barrier width are considered of 10 and 20 nm respectively. Since, the value of barrier height is obtained as 0.126 eV, the energy is varied up to 0.1 eV. The eigen energy value is obtained at 0.04 eV for a single quantum well for this particular structure. From this result, it can be shown that the resonant transmission peak is appeared at 0.04 eV. The other peaks are diminished at the rest of the energy positions.

The variation of tunneling current (J_T) with increasing bias voltage (V_bias) is shown in Fig. 3 for single quantum well. As the experimental data are unavailable for the proposed model, the analytical result obtained from this work is compared with the data obtained from the relevant model of strain free GeSn based RTD proposed by Wu et al.^[18]. From the comparison it is observed that with the structure proposed in this work a large tunneling current density is achieved in order of 450 kA/cm² with a band offset of 126 meV whereas with the strain-free GeSn based RTD the tunneling current of 300 kA/cm² is obtained at 48.3 meV of band offset. The tunneling current density is dependent on the transmission coefficient. From Eq. (19), it is clear that the value of ${J_{\rm{T}}}$ is increased with the different peaks of the parameter $\tau ({E_{\rm{x}}})$ . For higher transmission coefficient, the current density becomes higher. So, the curve of Fig. 3 is higher than the reported results by Wu et al.^[18]. The sharp decrease of peak current density occurred due to 0.75% tensile strain. After a certain change of peak current density, the tunneling current density is again enhanced at a value of 160 kA/cm².

The tunneling current density is also plotted in Fig. 4 against the energy of the uniform MQW structure with the number of quantum wells (n) as a parameter. It is found that the transmission peak is one for a single well and it is different for the double and triple well structure within a broader range of energy. These peaks indicate the presence of resonance in the well-barrier structure. Since, the tunneling is always dependent on transmission; the highest resonant transmission peak is observed almost at the eigen energy value of 0.04 eV for different numbers of wells.

The effect of increment in bias voltage on tunneling current density for MQW is displayed in Fig. 5. All the curves in Fig. 5 indicate that the peak of current density is reached at ~450 kA/cm². For a different number of quantum wells (n), the maximum values of PCD are different. It is also noted that for a double well, the NDR region is changed twice. If the number of wells is three, it is occurs thrice and so on. So, both the PCD and NDR region are changed regarding well manner for MQW, which gives an ON–OFF state in switching applications.

4. Conclusions

In this work a MQW GeC based RTD structure is studied and mainly the tunneling current density of the structure is estimated. It has been observed, in this study, that the calculated values of tunneling current density are in the order of magnitudes higher than the reported values of RTD structure using Si_1−x−yGe_xSn_y/Ge_0.9Sn_0.1/Si_1−x−yGe_xSn_y reported in Ref. [18]. The effect of NDR is also very significant in this discussion at room temperature (300 K). So, the possibility of the power dissipation becomes low and the speed of the device becomes higher. It is expected that this would give a good potentiality of the design of a binary and ternary adder. The huge change of NDR in MQW gives a high quality switching, amplification and oscillation. It can be concluded that the current applications lead to the possibility of achieving the ultra-high-speed digital devices and high frequency oscillators in the near future.

Acknowledgements

The second author (VC) acknowledges supports from B.P. Poddar Institute of Management and Technology (ECE Dept), Kolkata. The first author (SD) acknowledges support by TEQIP-Phase III under University College of Technology-Calcutta University (UCT-CU) through award of a fellowship.